Generalized fracturing activation criteria
-
摘要: 岩石破裂及其后续活动是最基本的构造变形方式，然而，经典的破裂准则（Coulomb-Mohr准则、Griffith准则和以Byerlee律为代表的滑动摩擦律）在实际应用中存在不同方面的局限。文章在经典破裂准则的基础上，从产生破裂（扩张破裂和剪切破裂）的物理本质出发，结合广义剪切活动准则和长期的研究实践，通过理论分析，提出了“广义破裂活动准则”。该准则用统一的无量纲因子（破裂活动趋势因子）来表达扩张破裂活动和剪切破裂活动，可以用来定量判断任意介质、在任意三轴应力状态下、任意方位界面（包括先存薄弱面和非薄弱面）发生破裂活动的可能性以及破裂的类型，把Coulomb-Mohr准则、Byerlee律和Griffith准则统一起来，将破裂（fracturing）扩展到破裂活动（fracturing activity）。该准则在与破裂活动相关的资源勘探开发（如页岩气、干热岩等）、灾害（如地震与滑坡等）预测和防治中具有广阔的应用前景。
-
关键词:
- 破裂 /
- 破裂活动 /
- 先存构造 /
- Coulomb-Mohr准则 /
- Byerlee律 /
- Griffith准则 /
- 广义破裂活动准则
Abstract:Objective Rock fracturing and its subsequent activations are the most basic tectonic deformation modes. However, the classical fracturing criteria (Coulomb-Mohr criterion, Griffith criterion, and Byerlee sliding-friction law) have different limitations in practical applications. Methods Based on the classical fracturing criteria and the analysis of the physical nature of fracturing generation (extensional fracturing and shear fracturing), combined with the generalized shear activation criterion and long-term research practice, a "generalized fracturing activation criterion" is proposed through theoretical analysis in this paper. Conclusion This criterion can be used to quantitatively determine the possibility and types of fracturing of any medium, under any triaxial stress state, and at any orientation interface (including pre-existing weak surface and non-weakness surface). It unifies the Coulomb-Mohr criterion, Byerlee's law, and Griffith's criterion, and extends fracturing to fracturing activation. Significance The proposed criterion has broad application prospects in the fracturing activation-related resource (such as shale gas and hot, dry rock) exploration and development and prediction and prevention of natural disasters (such as earthquakes and landslides). -
图 1 Coulomb-Mohr准则与Griffith准则图解
φ为岩石的内摩擦角；T为岩石的抗张强度；内聚力C=2T
Figure 1. Diagram of Coulomb-Mohr criterion and Griffith criterion
φ is the internal friction angle of the rock, T is the tensile strength of the rock and the cohesion C=2T
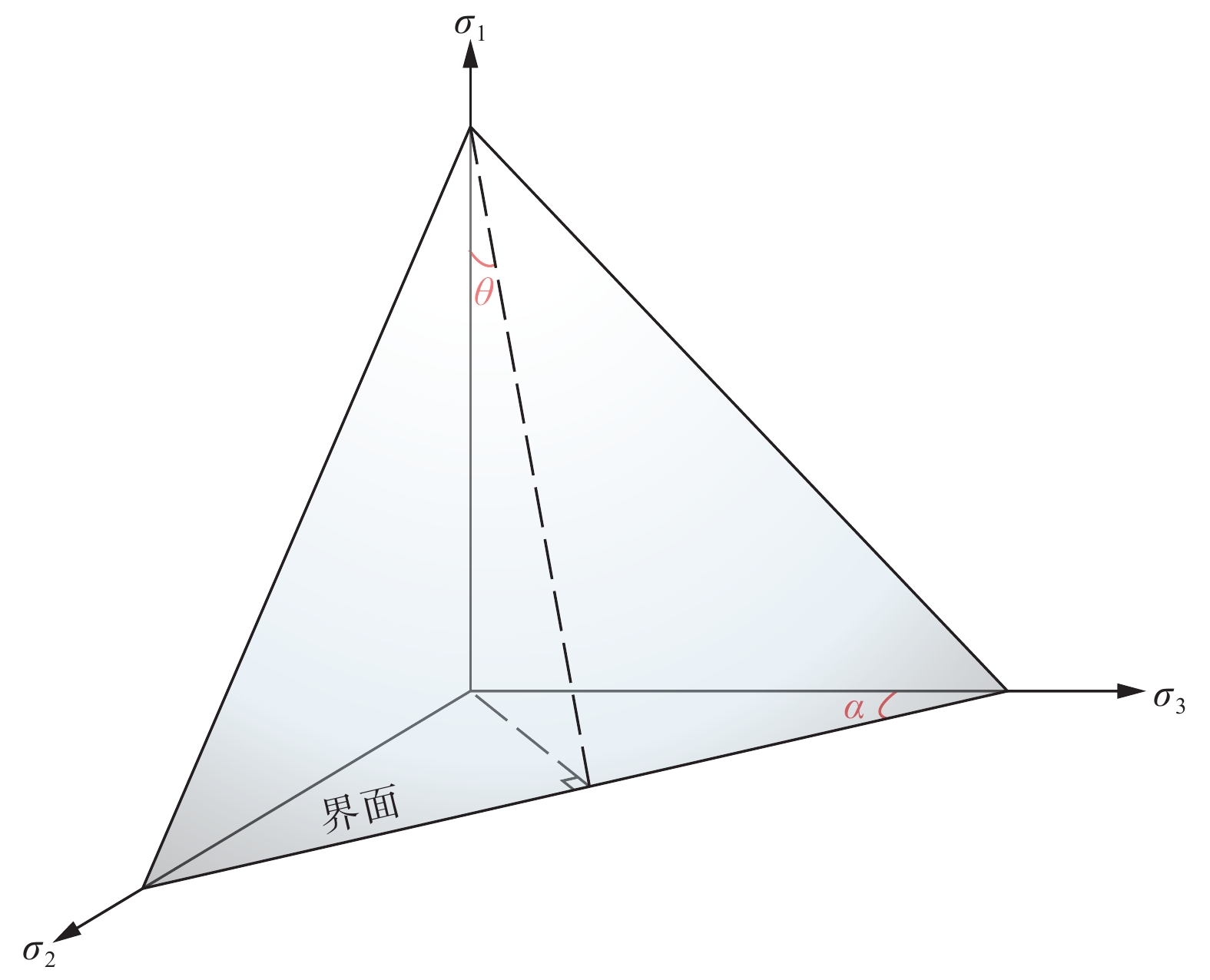
图 2 界面的空间方位定义（以3个主应力为坐标轴）
σ1、σ2和σ3分别为最大、中间和最小主应力；θ为界面与σ1的夹角；α为界面在σ2−σ3平面上的交线与σ3的夹角
Figure 2. The spatial orientation definition of the interface (with 3 principal stresses as axes)
σ1, σ2 and σ3 are the maximum, intermediate and minimum principal stresses respectively, θ is the angle between the interface and σ1, and α is the angle between σ3 and the interface's intersection line with the σ2–σ3 plane.
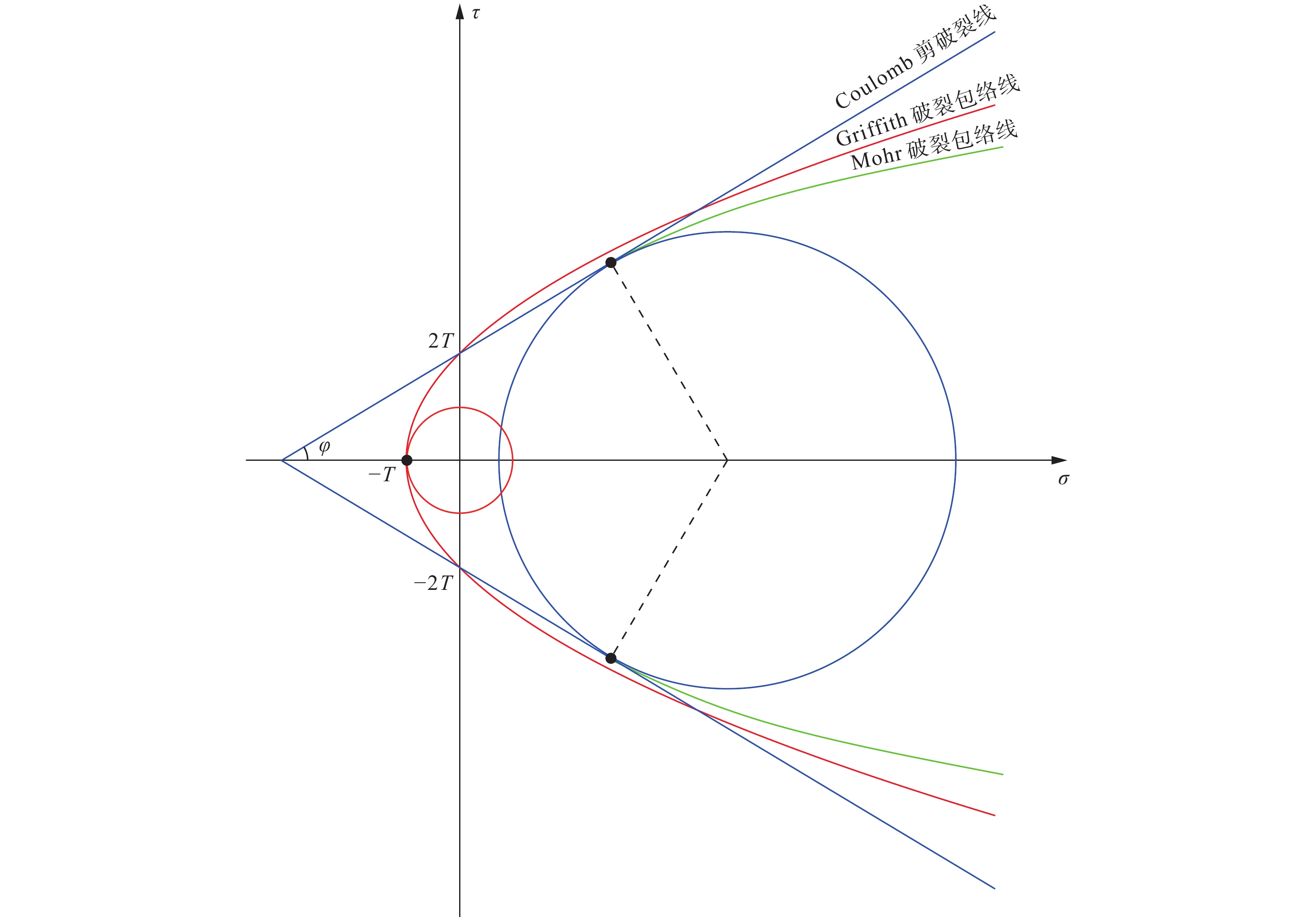
图 3 Griffith应力状态下的摩尔空间图解
T为岩石的抗张强度；2T为岩石的内聚力；TP为薄弱面的扩张强度；g为Griffith张破裂线；gP为薄弱面的扩张活动线；θ 为界面与 σ 1的夹角； α 为界面在 σ 2− σ 3平面上的交线与 σ 3的夹角
Figure 3. Diagram of the Mohr space under Griffith stress state
T is the tensile strength of the rock, 2T is the cohesion of the rock, TP is the extension strength of the weakness plane, g is the Griffith tensile fracture line, gP is the extension activation line of the weakness plane, θ is the angle between the interface and σ 1, and α is the angle between σ 3 and the interface's intersection line with the σ 2- σ 3 plane.
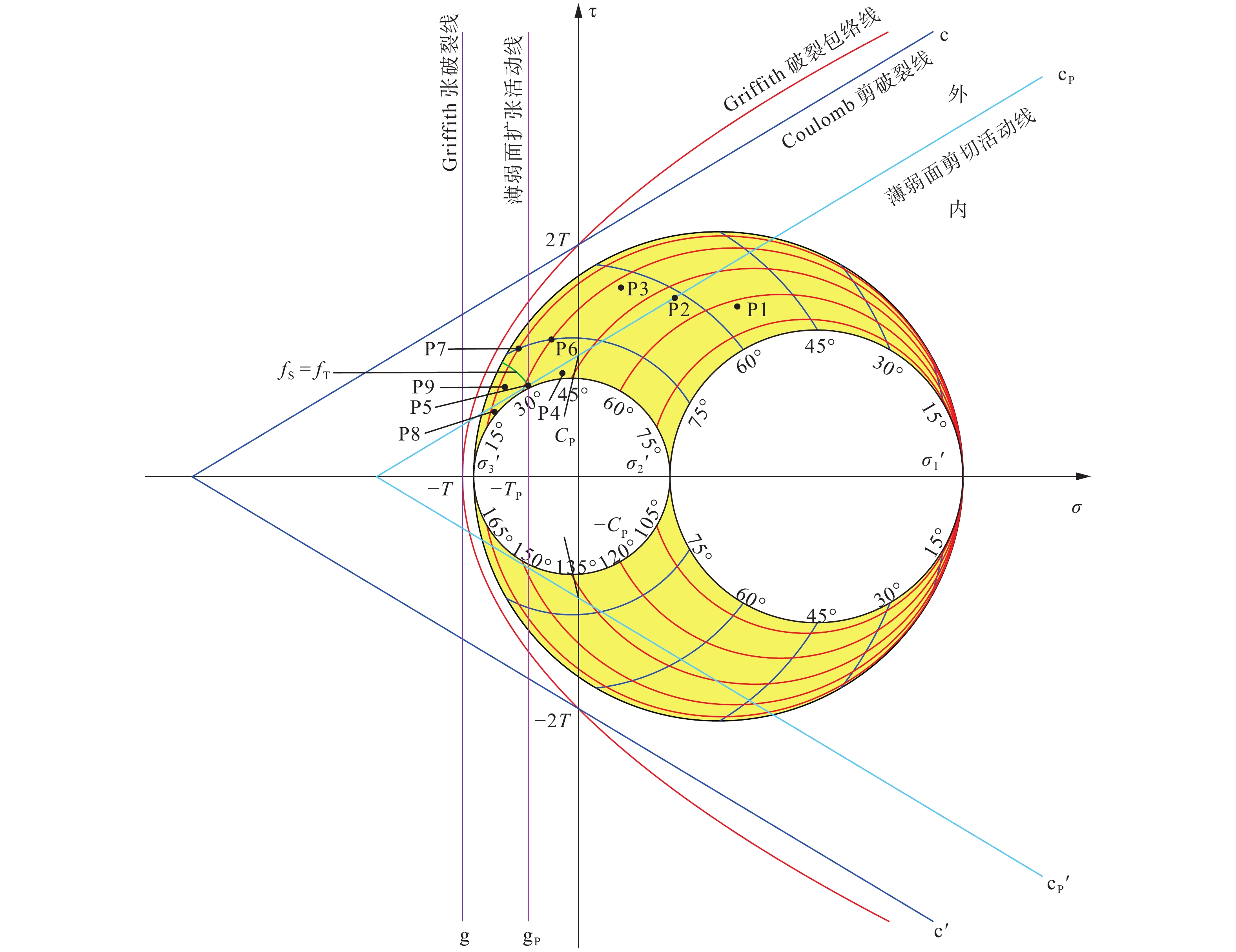
图 4 给定应力状态下摩尔空间中不同极点与破裂活动线的关系图解
T为岩石的抗张强度；2T为岩石的内聚力；C 为介质的内聚力；C P为界面的内聚力TP为薄弱面的扩张强度；g为Griffith张破裂线；gP为薄弱面的扩张活动线；P1—P9为不同薄弱面在摩尔空间中的极点位置
Figure 4. Diagram of relationship between different poles and fracturing activity line in Mohr space under given stress state
T is the tensile strength of the rock, 2T is the cohesion of the rock, C is the cohesion of the medium, CP is the cohesion of the interface, TP is the extension strength of the weakness plane, g is the Griffith tensile fracture line, gP is the extension activation line of the weakness plane, and P1–P9 are the pole positions of different weakness planes in Mohr-space.
图 5 含多个薄弱面地质体在流体压力不断增大过程中扩张活动演化的摩尔空间图解
T—岩石的抗张强度；2T—岩石的内聚力；TP—薄弱面的扩张强度；g—Griffith张破裂线；gP—薄弱面的扩张活动线a—初始应力状态下的摩尔空间图解；b—极点P1与扩张活动线接触应力状态下的摩尔空间图解；c—极点P2与扩张活动线接触应力状态下的摩尔空间图解；d—Griffith应力状态下的摩尔空间图解；e—应力降后区域应力状态下的摩尔空间图解
Figure 5. Mohr space diagram of the evolution of extension activities in geological bodies with multiple weak surfaces in the process of increasing fluid pressure
(a) Mohr space diagram in the initial stress state; (b) Mohr space diagram under the stress state when pole P1 touches the extension activation line; (c) Mohr space diagram under the stress state when pole P2 touches the extension activation line; (d) Mohr space diagram under Griffith stress state; (e) Mohr space diagram of regional stress state after stress drop T is the tensile strength of the rock, 2T is the cohesion of the rock, TP is the extension strength of the weakness plane, g is the Griffith tensile fracture line, and gP is the extension activation line of the weakness plane.
-
[1] ANDERSON E M, 1951. The dynamics of faultingand dyke formation with applications to Britain[M]. 2nd ed. Edinburgh: Oliver and Boyd. [2] BAILEY I W, BEN-ZION Y, 2009. Statistics of earthquake stress drops on a heterogeneous fault in an elastic half-space[J]. Bulletin of the Seismological Society of America, 99(3): 1786-1800. doi: 10.1785/0120080254 [3] BOTT M H P, 1959. The mechanics of oblique slip faulting[J]. Geological Magazine, 96(2): 109-117. doi: 10.1017/S0016756800059987 [4] BYERLEE J, 1978. Friction of rocks[J]. Pure and AppliedGeophysics, 116(4-5): 615-626. [5] CÉLÉRIER B, 2008. Seeking Anderson’s faulting in seismicity: a centennial celebration[J]. Reviews of Geophysics, 46(4): RG4001. [6] GRIFFITH A A, 1921. The phenomena of rupture and flow in solids[J]. Philosophical Transactions of the Royal SocietyA: Mathematical, Physical and Engineering Sciences, 221(582-593): 163-198. [7] GUDMUNDSSON A, SIMMENES T H, LARSEN B, et al. , 2010. Effects of internal structure and local stresses on fracture propagation, deflection, and arrest in fault zones[J]. Journal of Structural Geology, 32(11): 1643-1655. doi: 10.1016/j.jsg.2009.08.013 [8] JAEGER J C, COOK N G W, 1979. Fundamentals of rock mechanics[M]. 3rd ed. London: Chapman and Hall. [9] MCKENZIE D P, 1969. The relation between fault plane solutions for earthquakes and the directions of the principal stresses[J]. Bulletin of the Seismological Society of America, 59(2): 591-601. doi: 10.1785/BSSA0590020591 [10] MORLEY C K, GABDI S, SEUSUTTHIYAK, 2007. Fault superimposition and linkage resulting from stress changes during rifting: examples from 3D seismic data, Phitsanulok Basin, Thailand[J]. Journal of Structural Geology, 29(4): 646-663. doi: 10.1016/j.jsg.2006.11.005 [11] MORRIS A, FERRILL D A, HENDERSON D B, 1996. Slip-tendency analysis and fault reactivation[J]. Geology, 24(3): 275-278. doi: 10.1130/0091-7613(1996)024<0275:STAAFR>2.3.CO;2 [12] TONG H M, MENG L J, CAI D S, et al. , 2009. Fault formation and evolution in rift basins: sandbox modeling and cognition[J]. Acta Geologica Sinica, 83(6): 759-774. (in Chinese with English abstract) [13] TONG H M, CAI D S, WU Y P, et al. , 2010. Activity criterion of pre-existing fabrics in non-homogeneous deformation domain[J]. Science China Earth Sciences, 53(8): 1115-1125. doi: 10.1007/s11430-010-3080-6 [14] TONG H M, YIN A, 2011. Reactivation tendency analysis: a theory for predicting the temporal evolution of preexisting weakness under uniform stress state[J]. Tectonophysics, 503(3-4): 195-200. doi: 10.1016/j.tecto.2011.02.012 [15] TONG H M, KOYI H, HUANG S, et al. , 2014. The effect of multiple pre-existing weaknesses on formation and evolution of faults in extended sandbox models[J]. Tectonophysics, 626: 197-212. doi: 10.1016/j.tecto.2014.04.046 [16] TONG H M, WANG J J, ZHAO H T, et al. , 2014. Mohr space and its application to the activation prediction of pre-existing weakness[J]. Science China Earth Sciences, 57(7): 1595-1604. doi: 10.1007/s11430-014-4860-1 [17] TONG H M, CHEN Z L, LIU R X, 2015. Generalized shear activation criterion[J]. Chinese Journal of Nature, 37(6): 441-447. (in Chinese with English abstract) [18] TONG H M, LIU Z P, ZHANG H X, et al. , 2021. Theory and method of temporary macrofracture plugging to prevent casing deformation in shale gas horizontal wells[J]. Natural Gas Industry, 41(5): 92-100. (in Chinese with English abstract) [19] TONG H M, ZHANG P, ZHANG H X, et al. , 2021. Geomechanical mechanisms and prevention countermeasures of casing deformation in shale gas horizontal wells[J]. Natural Gas Industry, 41(1): 189-197. (in Chinese with English abstract) [20] TWISS R J, MOORES E M, 1992. Structural geology[M]. San Francisco: W. H. Freeman & Co. : 532. [21] WALLACE R E, 1951. Geometry of shearing stress and relation to faulting[J]. The Journal of Geology, 59(2): 118-130. doi: 10.1086/625831 [22] XU K L, ZHUZ C, 1989. Structural geology[M]. 2nd ed. Beijing: Geology Press: 270. (in Chinese) [23] YIN A, 1994. Mechanics of monoclinal systems in the Colorado plateau during the Laramide orogeny[J]. Journal of Geophysical Research: Solid Earth, 99(B11): 22043-22058. doi: 10.1029/94JB01408 [24] ZOBACK M D, 2007. Reservoir geomechanics[M]. New York: Cambridge University Press. [25] 童亨茂, 孟令箭, 蔡东升, 等, 2009. 裂陷盆地断层的形成和演化: 目标砂箱模拟实验与认识[J]. 地质学报, 83（6）: 759-774. doi: 10.3321/j.issn:0001-5717.2009.06.002 [26] 童亨茂, 王建君, 赵海涛, 等, 2014. “摩尔空间”及其在先存构造活动性预测中的应用[J]. 中国科学: 地球科学, 44（9）: 1948-1957. [27] 童亨茂, 陈正乐, 刘瑞珣, 2015. 广义剪切活动准则[J]. 自然杂志, 37（6）: 441-447. [28] 童亨茂, 刘子平, 张宏祥, 等, 2021a. 暂堵大裂缝防治页岩气水平井套管变形的理论与方法[J]. 天然气工业, 41（5）: 92-100. [29] 童亨茂, 张平, 张宏祥, 等, 2021b. 页岩气水平井开发套管变形的地质力学机理及其防治对策[J]. 天然气工业, 41（1）: 189-197. [30] 徐开礼, 朱志澄, 1989. 构造地质学[M]. 2版. 北京: 地质出版社: 270. -
下载:
计量
- 文章访问数: 543
- HTML全文浏览量: 158
- PDF下载量: 119
- 被引次数: 0
