基于FEMM-Fracflow研究缝洞型油藏中裂缝扩展问题

王慧; 刘泉声

doi:10.12090/j.issn.1006-6616.2020.26.01.006

基于FEMM-Fracflow研究缝洞型油藏中裂缝扩展问题

doi: 10.12090/j.issn.1006-6616.2020.26.01.006

王慧,
刘泉声^,

武汉大学土木建筑工程学院, 湖北武汉 430072

基金项目:

国家自然科学基金 41602296

详细信息

作者简介:
王慧(1994-), 女, 在读硕士, 研究方向为多物理场裂缝扩展问题。E-mail:huiwangwhu@163.com

通讯作者:
刘泉声(1962-), 男, 教授, 从事岩石力学方面研究。E-mail:2992464906@qq.com

中图分类号: P618.13
计量
- 文章访问数: 363
- HTML全文浏览量: 123
- PDF下载量: 17
- 被引次数: 0
出版历程
- 收稿日期: 2019-01-11
- 修回日期: 2019-06-16
- 刊出日期: 2020-02-29

Investigation on fracture propagation in fractured-cavity reservoirs based on FEMM-fracflow modelling

WANG Hui,
LIU Quansheng^,

School of Civil Engineering, Wuhan University, Wuhan 430072, Hubei, China

摘要

摘要: 在缝洞型油藏中，水力裂缝的偏转路径对石油的开采量有重要的影响。Hybrid Finite-element and Mesh-free Method-Fracflow（FEMM-Fracflow）数值模拟平台，通过数值实验，文章分析了缝洞型油藏中自然溶洞、水平地应力以及注水流速三种因素对水力裂缝偏转路径的影响。结果表明，在存在溶洞时，裂缝明显向溶洞方向偏转；在改变水平围压时，不施加水平围压条件下，裂缝明显偏向溶洞方向扩展，并且最终与溶洞连通；而在施加50 MPa水平围压时，水力裂缝偏向溶洞的趋势明显减弱；在改变流速时，当流速为0.05 kg/s，裂缝明显向溶洞方向偏转，而当流速为0.2 kg/s，裂缝向溶洞方向偏转的趋势则减弱。
- 缝洞型油藏 /
- 水力压裂 /
- FEMM-Fracflow /
- 流固耦合 /
- 连通性
Abstract: The propagation path of hydraulic fractures is critical to oil recovery in fractured-cavity reservoirs. Based on Hybrid Finite-element and Mesh-free Method-Fracflow (FEMM-Fracflow) numerical simulation platform, this paper explores the influence of natural caves, in-situ stress and injection velocity on the propagation path of hydraulic fractures in reservoirs. The simulation results show that when there are caves, the fracture propagates toward the cave. When the horizontal confining pressure is changed, the fracture propagates toward the cave obviously without applying horizontal confining pressure, and eventually connects with the cave; when the horizontal confining pressure of 50 MPa is applied, the trend of hydraulic fracture propagating toward the cave is obviously weakened; when injection velocity is changed, the fracture propagates toward the cave with the injection velocity of 0.05 kg/s, while the tendency of fracture propagating toward the cave is weakened with the injection velocity of 0.2 kg/s.
- fractured-cavity reservoir /
- hydraulic fracturing /
- FEMM-Fracflow /
- fluid-structure coupling /
- connectivity
注释:

责任编辑：范二平

HTML全文

0. 引言

缝洞型油藏在自然界广泛分布(Khvatova et al., 2012; 祖克威等，2018)，这种油藏中包含着大量的从厘米级到米级的溶洞，这造成了缝洞型油藏很强的地质复杂性(Zhao et al., 2015; 王璐等，2017)。在这种油藏中，溶洞是主要的储油空间(Gao et al., 2016)。近年来，水力压裂技术(谢军等，2015；付金伟等，2017)被广泛应用于水利工程、岩土工程、地热开发和石油工程等领域，许多学者对不同条件下的水压致裂机理、裂纹扩展机理进行了研究。在室内试验方面，杨潇等(2017)研究了水力裂缝扩展过程中裂缝宽度的变化过程；武鹏飞等(2017)对煤岩复合体水压致裂裂缝扩展规律进行了试验研究；赵子江等(2019)研究了注水方式(循环注水和一次性注水)对水力压裂的影响。在数值模拟方面，龚迪光等(2016)采用ABAQUS研究了水平应力差、注水速度和液体粘度对水力压裂过程的影响；刘国威等(2017)提出了相场模拟方法，为水力压裂提供了一种新的数值模拟方法；姚池等(2017)提出了改进刚体弹簧方法模拟各向同性岩体水压致裂过程，并研究了Biot系数对水力压裂的影响；严成增等(2016)采用有限元离散元耦合数值模拟方法FDEM研究了地应力对水力压裂的影响。通过总结已有研究成果发现，无论是室内试验还是数值模拟，对各向同性、均质完整岩体或含天然小型裂隙(指开度较小)岩体的水力压裂研究较为丰富；对水力压裂过程、裂纹扩展机理和水力压裂影响因素的研究等也取得了丰富的成果。然而，对于天然大型溶洞(开度米级以上)对水力裂缝扩展路径的影响研究相对较少；而在石油工程中，除了小型裂隙、孔隙外，大型溶洞也是主要的储油空间，水力裂缝的扩展路径关系到能否从溶洞中顺利抽采石油，因此，对含大型溶洞岩体中水力裂缝扩展规律和影响因素的研究显得十分重要。水力裂缝的扩展路径受到多种因素的影响，如溶洞、围压(伍亚等，2014)和注入流速等。根据与溶洞的连接关系，水力裂缝可分为三种不同的类型：连接型裂缝，即裂缝与溶洞相连接；偏向型裂缝，即裂缝向溶洞方向偏转；偏离型裂缝，即裂缝向远离溶洞方向偏转。

有限元(Zienkiewicz and Taylor, 2000)是一种广泛使用的方法，但是在涉及到裂缝扩展(Adachi et al., 2007)问题时，有限元方法需要重新划分网格，三维结构网格的划分更加复杂。近些年来，一系列可以用来模拟裂缝扩展的数值方法相继提出，如无网格法(Rajendran and Zhang, 2007)、基于单位分解法(Melenk Babuška，1996)的广义有限元法(Strouboulis et al., 2000)、扩展有限元法(郝志勇和岳立新，2018)、有限差分法(Belytschko et al., 2009; 王雄等，2017)和数值流形法(Shi，1991)等。这些方法通过构造高阶权函数，而不用增加额外的节点，因此具有较高的精度和收敛率。对于无网格法而言，其近似函数建立在一系列离散点上，不需要借助于网格，克服了有限元对网格的依赖性。由于不需要用网格离散计算区域，可以用来解决流固耦合问题。为了利用不同数值方法的优点, Rajendran and Zhang(2008)结合了无网格法和有限元法的思想，提出了杂交无网格-有限元法FEMM，这种方法不需要重新划分网格，具有较高的精度，可以用来模拟岩体中的裂缝扩展问题。随后，在Rajendran and Zhang工作的基础上，学者们相继提出了基于连续节点应力的四节点四边形单元(Tang et al., 2009)和三节点三角形单元(Yang et al., 2014)用来分析裂缝扩展问题，如Liu et al.(2018)利用FEMM模拟三维裂缝扩展问题。

水力压裂过程是一个典型的流固耦合过程。文章基于Liu et al.(2018)提出的流固耦合数值方法模拟水力压裂过程。岩石块体变形和裂缝扩展采用固体部分计算(FEMM)，该方法基于单位分解法，使用有限元形函数构造单位分解函数，同时该方法不需要重新划分网格，能够克服对网格的依赖性；而流体的流动过程采用流体部分计算(文中命名为Fracflow)，其基本原理是基于平行板模型(Witherspoon et al., 1980)的立方定律。岩石变形和裂缝扩展会影响流体的流动，而流体的流动反过来促进裂缝的扩展，二者相互耦合。

1. FEMM-Fracflow基本理论

1.1 FEMM基本理论

1.1.1 单位分解函数的形成

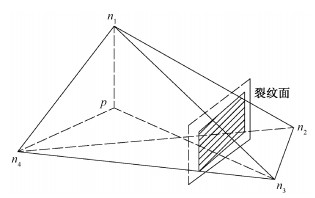
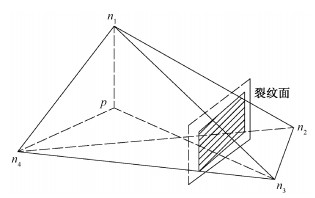
考虑任意一个四面体单元Ω，其四个顶点为{n₁, n₂, n₃, n₄}，如图 1所示。p(x)={x, y, z}代表四面体中的任意一点。根据单位分解法的原理，位移函数u(x)可以表示为：

图 1 被裂缝平面穿过的四面体

Figure 1. Tetrahedral element passed by a planar fracture

下载: 全尺寸图片幻灯片

$u(x) = \sum\limits_{i = 1}^4 {{w_i}} (x){u_i}(x)$

(1)

其中，w_i(x)是节点的非零单位分解函数，u_i(x)是节点的位移，单位分解函数满足单位分解条件：

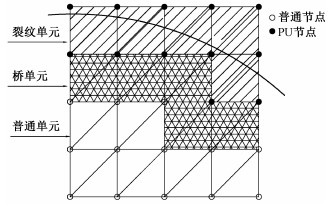
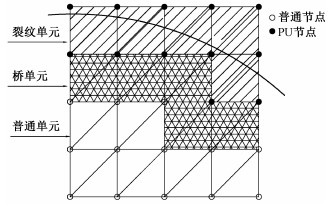
$\sum\nolimits_{i = 1}^4 {{w_i}(x)} = 1$

(2)

为方便覆盖域的描述，这里选取二维平面进行说明。如图 2所示，网格中存在3种单元：①裂缝单元——被裂缝穿过的单元；②桥单元——与裂缝单元有公共节点的单元；③普通单元——其余单元。裂缝单元上所有的节点为PU节点，其他节点为普通的有限元节点。桥单元中既有PU节点，也有普通有限元单元。

图 2 网格覆盖域中裂缝单元、桥单元和普通单元示意图

Figure 2. Schematic of the FE, bridge, fracture elements

下载: 全尺寸图片幻灯片

构造单位分解函数的方法很多，包括MLS形函数、有限元形函数和SHEPARD函数等。文中选择SHEPARD函数构造各覆盖区域的单位分解函数。共享该节点的所有单元构成一个覆盖，该覆盖中所有单元的权函数取有限元形函数。则单位分解函数可以写为：

${w_i}(x) = \frac{{{N_i}(x)}}{{\sum\nolimits_i {{N_i}(x)} }} = {N_i}(x),i \in I$

(3)

其中，N_i(x)是节点i对应的有限元法的形函数，分别为：

$\begin{array}{*{20}{c}} {{N_1}(x) = \frac{{{V_{{n_2}{n_3}{n_4}p}}}}{{{V_{{n_1}{n_2}{n_3}{n_4}}}}}}\\ {{N_2}(x) = \frac{{{V_{{n_3}{n_4}{n_1}p}}}}{{{V_{{n_1}{n_2}{n_3}{n_4}}}}}}\\ {{N_3}(x) = \frac{{{V_{{n_4}{n_1}{n_2}p}}}}{{{V_{{n_1}{n_2}{n_3}{n_4}}}}}} \end{array}$

(4)

其中，V_{n_in_jn_kp}是点n_i, n_j, n_k, p组成的四面体的体积。

1.1.2 裂缝单元近似函数构造

当某单元被裂缝穿过时，裂缝两侧为不连续位移场，因此该单元的单位分解函数有所不同，此时应该对权函数进行修正。定义I_τ是该单元区域中节点索引集合，基于可视化准则构造不连续位移场，定义可视化区域 $I_{\tau}^{\mathrm{vis}}(x) \subset I_{\tau}$ 。

$I_\tau ^{{\rm{vis}}}(x) = \left\{ {i \in {I_\tau }|\left[ {x - {x_i}} \right] \cap f = \emptyset } \right\}$

(5)

其中，x_i是节点n_i的坐标，[x-x_i]表示连接这两点的线段。

则修正后的权函数为：

${{\alpha '}_i}(x) = \left\{ {\begin{array}{*{20}{l}} {\frac{{{N_i}(x)}}{{\sum\nolimits_i {{N_i}(x)} }}}&{i \in I_\tau ^{{\rm{vis}}}(x)}\\ 0&{i \notin I_\tau ^{{\rm{vis}}}(x)} \end{array}} \right.$

(6)

则在裂缝单元上，修正后的单位分解函数为：

$w_i^\prime (x) = \left\{ {\begin{array}{*{20}{l}} {\frac{{\alpha _i^\prime (x)}}{{\alpha _1^\prime (x) + \alpha _2^\prime (x) + \alpha _3^\prime (x) + \alpha _4^\prime (x)}}}&{i \in I_\tau ^{{\rm{vis}}}(x)}\\ 0&{i \notin I_\tau ^{{\rm{vis}}}(x)} \end{array}} \right.$

(7)

1.1.3 岩体本构关系及裂缝扩展条件

该文假设岩体为各项同性，岩体应力应变行为符合弹性关系，同时文章采用最大拉应力准则判断裂缝是否扩展，公式如下：

${\sigma ^{{\rm{tip}}}} = {T_0}$

(8)

其中，σ^tip是裂缝尖端的最大拉应力，T₀是岩体的抗拉强度。当最大拉应力值超过抗拉强度时，裂缝便会垂直于最大拉应力方向扩展。

1.2 Fracflow基本理论

1.2.1 流体计算控制方程

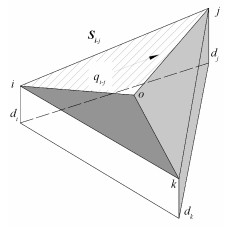
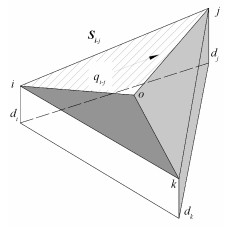
裂缝单元中的流动假定为平行板流。节点i的影响区域是跟节点相连的所有裂缝单元。如图 3所示，选取任意一个裂缝单元V_Δijkⁱ，o是裂缝单元的中心点，裂缝单元被中心点o分为三个子区域。S_i-j为节点i和节点j之间的水流路径。假设水压呈线性分布，则流动路径上的压力梯度为：

图 3 裂缝单元流体流动路径

Figure 3. Flow path associated with a fracture-element

下载: 全尺寸图片幻灯片

${J_{i - j}} = \frac{{{p_i} - {p_j} + \rho g\left( {{z_i} - {z_j}} \right)}}{{{l_{i - j}}}}$

(9)

其中，P_i, p_j分别为节点i, j的水压；z_i, z_j分别是节点i和节点j竖坐标, l_i-j是节点i和节点j之间的距离，ρ为密度，g为重力加速度。

流体流速q计算如下：

${q_{i - j}} = \left( {\frac{{{f_{si}} + {f_{sj}}}}{2}} \right) \cdot \frac{{\delta _{i - j}^2{J_{i - j}}}}{{12\mu }} \cdot {b_{i - j}}$

(10)

${b_{i - j}} = \frac{{{S_{i - j}}}}{{{l_{i - j}}}}$

(11)

${f_s} = {s^2}(3 - 2s)\;\;\;\;0 \le s \le 1$

(12)

其中，s代表节点饱和度，μ是流体的黏度，b_i-j是节点i到节点j的流体路径的等效宽度，δ_i-j是等效开度。

1.2.2 流体压力的更新

在任意时步，流体的压力为：

${p^n} = \left\{ {\begin{array}{*{20}{l}} {{p^{n - 1}} + {K_{\rm{w}}}Q\frac{{\Delta t}}{{{V^n}}} - {K_{\rm{w}}}\frac{{\Delta V}}{{{V_m}}}}&{s = 1}\\ 0&{s < 1} \end{array}} \right.$

(13)

其中，pⁿ, p^n-1分别代表当前时步和上一时步的流体压力，K_w为流体的体积模量，Q为每秒总流量，为时间步增量，Vⁿ是当前时步节点的体积，V^n-1是上一时步节点的体积。ΔV、V_m分别为相邻时步之间节点体积差、相邻时步节点体积之和的一半，如下：

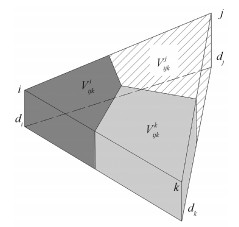
$\Delta V = {V^n} - {V^{n - 1}}$

(14)

${V_{\rm{m}}} = \frac{{{V^n} + {V^{n - 1}}}}{2}$

(15)

对于一个裂缝单元Δ_ijk，各节点对应的体积V_Δijkⁱ，如图 4所示。节点i的饱和度更新如下:

图 4 裂缝单元子区域体积定义

Figure 4. The volume of node associated with a fracture-element

下载: 全尺寸图片幻灯片

${s^n} = {s^{n - 1}} + Q\frac{{\Delta t}}{V} - \frac{{\Delta V}}{{{V_{\rm{m}}}}}$

(16)

其中，sⁿ和s^n-1分别代表当前时步和上一时步节点i的饱和度。

1.3 FEMM与Fracflow耦合

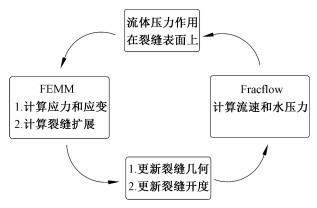
该文将固体计算模块FEMM与流体计算模块Fracflow耦合，用于求解缝洞型油藏中裂缝扩展的流固耦合问题，如图 5所示。具体步骤如下：①在FEMM中求解岩体的应力应变场，裂缝扩展方向及长度；②更新的裂缝方向和长度数据传递到Fracflow中，计算裂缝面上的水压分布；③计算所得水压传递到FEMM中，再次计算应力应变场，更新裂缝扩展方向及扩展长度。

图 5 FEMM与Fracflow耦合原理示意图

Figure 5. Scheme of coupling principle between FEMM and Fracflow

下载: 全尺寸图片幻灯片

2. 数值算例

2.1 裂缝开度计算验证

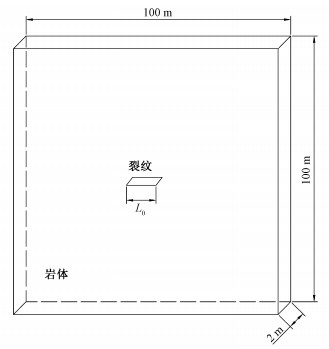
通过比较FEMM和ABAQUS的裂缝开度计算结果，验证数值平台中固体模块计算的有效性。数值模型的几何示意图如图 6所示，模型大小为100 m×100 m×2 m。模型正中间预制一条水平的裂缝，长17 m，宽2 m，模型六个表面采用位移约束，裂缝面上施加恒定的水压力10 kPa。模型其他的材料参数如表 1所示。在FEMM中，裂缝为一个厚度可当作0的平面，模型的网格划分与裂缝的位置和形态无关，而在ABAQUS中，裂缝附近的模型网格划分依赖于裂缝的存在。

图 6 裂缝开度验证模型几何示意图

Figure 6. A cubic rock with a central fracture

下载: 全尺寸图片幻灯片

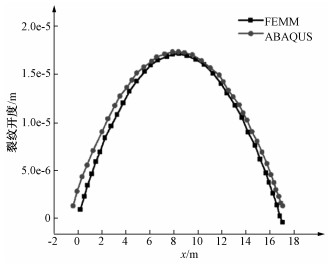
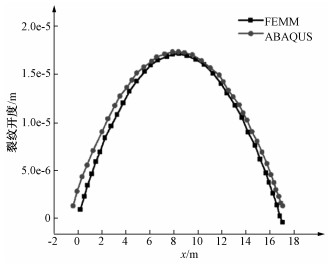
表 1 裂缝开度验证模型输入参数

Table 1. Input parameters for the cubic rock with a central fracture

输入参数	数值
杨氏模量E/GPa	10
泊松比v	0.25
恒定水压p/kPa	10

下载: 导出CSV

| 显示表格

为了定量地比较两者的计算结果，通过选取裂缝上表面的开度，绘制了图 7的拟合图。其中，在FEMM中，裂缝上表面的最大开度值为0.0177 m，在ABAQUS中，该值为0.0179 m, 两者的结果存在较小的误差，计算结果具有较好的一致性。

图 7 裂缝开度验证模拟结果

Figure 7. Simulation results of facture aperture

下载: 全尺寸图片幻灯片

2.2 裂缝扩展路径验证

在不考虑流体的情况下，模拟一个带空洞的平板中的裂缝扩展路径，并与Yang and Zhang(2016)的模拟结果进行比较。几何模型如图 8所示，模型的力学和材料参数如下：杨氏模量E=30 GPa，泊松比v=0.2，顶端拉力P=5 kPa，空洞半径d=0.20 m，空洞中心距离平板底端垂直距离D_f=1.50 m。一条人工裂缝位于平板左侧，初始长度为0.1 m。

图 8 带空洞平板几何图

Figure 8. Geometry of a thin plate with a hole and an edge fracture

下载: 全尺寸图片幻灯片

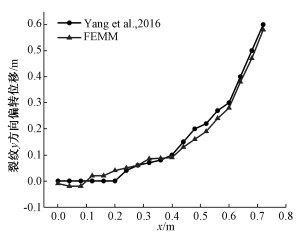
从裂缝扩展示意图可以看出(图 9)，裂缝逐渐偏向空洞方向扩展，直至与空洞连通。将文中裂缝沿y方向的偏转位移与文献中的偏转位移进行比较，如图 10所示，可以看到，两者结果具有较好的一致性。

图 9 带空洞平板裂纹偏转示意图

Figure 9. Fracture propagation near a hole without fluid

下载: 全尺寸图片幻灯片

图 10 裂缝沿y方向扩展位移比较

Figure 10. Comparison of the calculated path along the y direction for the fracture propagation near a hole without fluid with that of the reference

下载: 全尺寸图片幻灯片

2.3 溶洞对裂缝扩展的影响研究

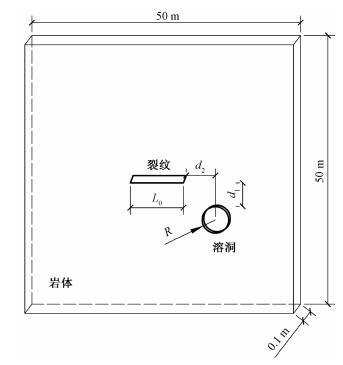
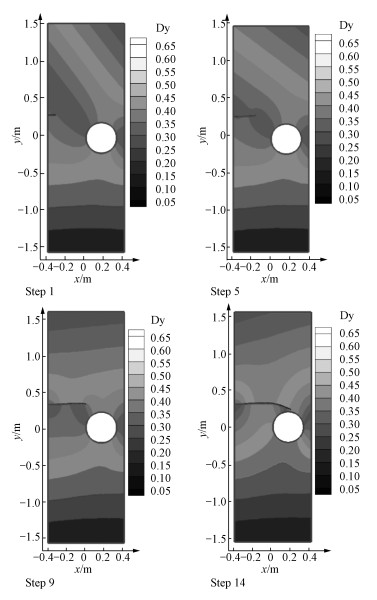
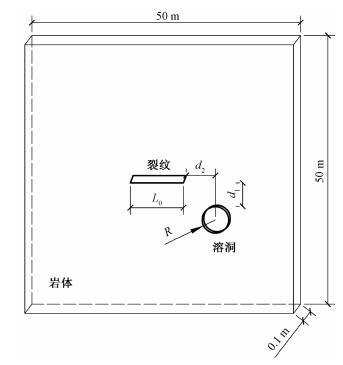
同时考虑流体流动的情况下模拟裂缝扩展路径，为了探究溶洞对裂缝扩展路径的影响规律，分别建立两个数值模型：完整岩体和带溶洞岩体，以比较两者裂缝扩展路径的区别。如图 11所示，模型几何尺寸为50 m×50 m×0.1 m，溶洞的半径为3 m。溶洞顶端与裂缝右侧的水平距离d₂=2 m，垂直距离d₁=2 m。模型1和模型2中裂缝面上注水速度恒定。模型1与模型2的材料参数均相同，如表 2所示。

图 11 带溶洞立方体几何图

Figure 11. A cubic domain with a cavity and a central fracture

下载: 全尺寸图片幻灯片

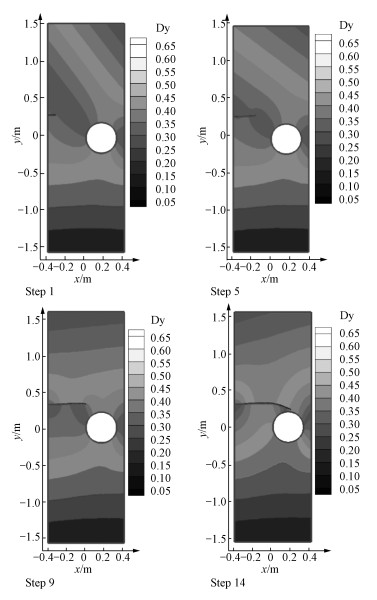
表 2 带溶洞模型输入参数

Table 2. Input parameters for a cubic domain

输入参数	值
杨氏模量E/GPa	9
泊松比v	0.25
抗拉强度f_t/MPa	1
密度ρ/(kg·m^-3)	1100
材料孔隙度	0.1
裂缝孔隙度	0.25
岩体渗透率/(m²·s^-1)	1.0×10^-20
裂缝渗透率/(m²·s^-1)	1.0×10^-10
流体黏度/(Pa·s^-1)	1.0×10^-3
流体注入速率/(kg·s^-1)	6.0×10^-2

下载: 导出CSV

| 显示表格

模型的竖向位移云图及裂缝偏转轨迹如图 12所示，在考虑渗流时，完整岩体的竖向变形是对称的，裂缝沿水平方向扩展，符合一般性规律。而存在溶洞的岩体，其位移场明显受到扰动，同时裂缝左侧基本沿水平方向扩展，而裂缝右侧由于应力集中，导致裂缝偏向溶洞方向扩展，最后与溶洞连通。由此可以看出，溶洞的存在会改变岩体中裂缝的偏转路径，并且会使裂缝偏向溶洞方向扩展。

图 12 岩体位移场分布

Figure 12. Vertical displacement distribution of the reservoir for hydraulic fracture propagation

下载: 全尺寸图片幻灯片

在对水力压裂的相关研究中发现，围压和注入流速是影响裂缝扩展路径的两个重要因素。基于此，下文将分别讨论围压和注入流速对缝洞型油藏中裂缝扩展路径的影响。

2.4 围压对缝洞型油藏中裂缝扩展路径影响

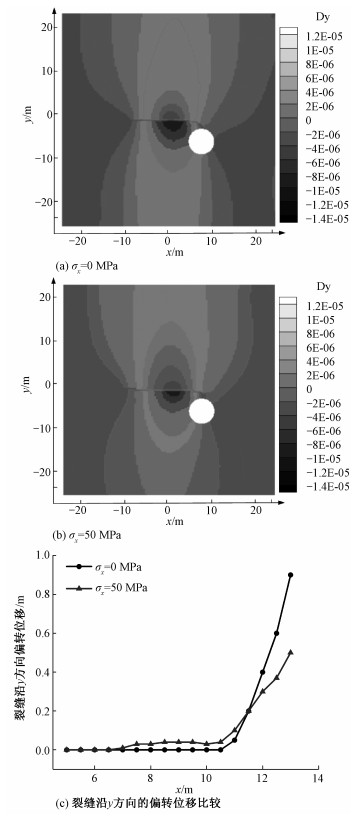
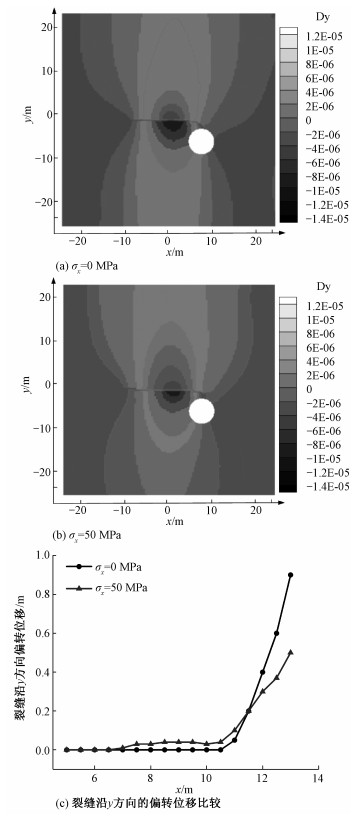
为探究不同围压对缝洞型油藏中裂缝扩展路径的影响，建立两个数值模型，模型尺寸与图 11相同。模型1中围压为0 MPa，模型2中围压为50 MPa，输入参数如表 2所示。

模型的竖向位移云图如图 13所示。从图 13a、13b中可以看出，由于溶洞的存在，裂缝右侧附近的应力场被改变，从而导致裂缝扩展路径的改变。然而随着围压的增大，溶洞对裂缝扩展路径的影响减弱。从13c可以看到，当岩体水平方向施加较大的围压时，裂缝沿y方向的位移较小，即裂缝偏向溶洞方向扩展的趋势减弱。因此，从数值模拟结果中可以看到，水平单向围压对缝洞型油藏水力裂缝扩展路径有重要影响，且随着围压的增大，裂缝由连接型裂缝转化为偏向型裂缝，说明围压增大时，对溶洞中石油的采出是不利的。

图 13 不同围压下岩体竖向位移图及裂缝沿y方向的偏转位移比较

Figure 13. Comparison of vertical displacement of the reservoir and fracture propagation path along the y direction under different confining pressures

下载: 全尺寸图片幻灯片

2.5 注水速度对缝洞型油藏中裂缝扩展路径影响

为了研究注水速度对缝洞型油藏中裂缝扩展路径的影响，分别采用了注水速度v=0.05 kg/s和v=0.2 kg/s的计算模型，得到如图 14所示的模拟结果。

图 14 不同注水速度下岩体竖向位移场分布

Figure 14. Vertical displacement distribution of the reservoir under different water injection velocities

下载: 全尺寸图片幻灯片

从图 14a、14b中可以看出，溶洞的存在改变了位移场的对称分布，裂缝右侧附近的应力场被改变，导致了裂缝扩展路径改变。从图 14c可以看出，当注水速度较小时，水力裂缝沿y方向的偏转位移更大，即水力裂缝偏向溶洞方向的趋势更强烈，而当注水速度增加时，裂缝沿y方向的偏转位移较小，即偏向溶洞方向扩展的趋势减弱，即注水速度越大，溶洞对水力裂缝扩展路径的影响越弱，水力裂缝由连接型裂缝转化为偏向型裂缝。因此，为了能从溶洞中顺利采出石油，不宜采用很大的注水速度，因为大的注水速度可能导致水力裂缝无法与溶洞连通。

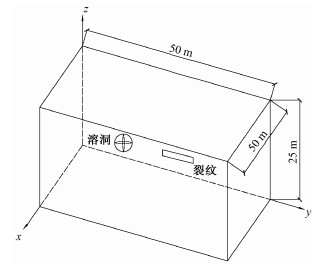
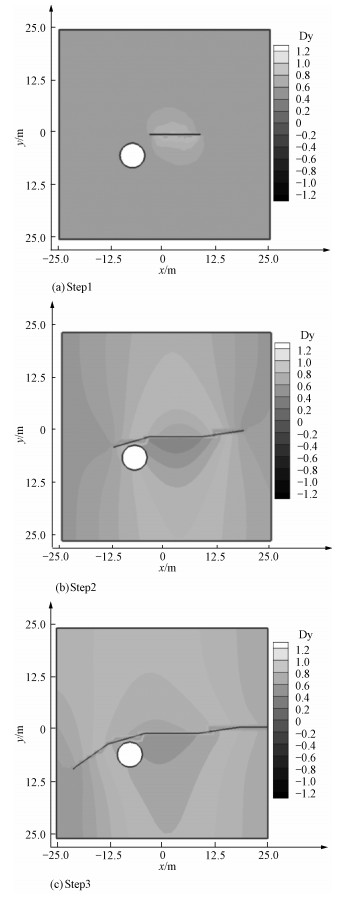
2.6 真三维球体溶洞对裂缝扩展路径的影响

为了反映工程中的实际情况，即溶洞储集空间通常是一个三维的封闭空间，试考虑真三维球体溶洞对裂缝扩展路径的影响，如图 15所示，模型的几何尺寸为xy平面为50 m×50 m，z平面为25 m。模型中心预制一条人工裂缝，长为25 m，宽为11 m。溶洞在岩体的右下部分，半径为3 m。岩体的8个角点在3个方向的位移为0，裂缝面上同样施加恒定水压力P=100 kPa。其他相关参数见表 3。数值模拟结果如图 16所示。从该图可以看出，远离溶洞一侧的裂缝基本沿水平方向偏转，而靠近溶洞一侧的裂缝却沿偏向溶洞的方向偏转，说明溶洞的存在干扰了裂缝附近的应力场和位移场，影响了水力裂缝的偏转轨迹。这样的偏转规律可以为缝洞型油藏的开采起到一定的指导作用。

图 15 缝洞型油藏三维模型

Figure 15. Geometry of the 3D model of the fractured-cavity reserior

下载: 全尺寸图片幻灯片

表 3 三维模型输入参数

Table 3. Input parameters for the 3D model

输入参数	值
杨氏模量E/GPa	1
泊松比v	0.20
抗拉强度f_t/MPa	1
密度ρ/(kg·m^-3)	1100
岩体孔隙度	0.06
岩体渗透率/m²	1.0×10^-20
裂缝处渗透率/m²	1.0×10^-10
黏度/(Pa·s^-1)	1.0×10^-3

下载: 导出CSV

| 显示表格

图 16 三维缝洞型油藏裂缝偏转示意图

Figure 16. Fracture propagation for the 3D hydraulic fracture propagation near a spherical cavity

下载: 全尺寸图片幻灯片

3. 结论

文章在FEMM-Fracflow数值平台的基础上，研究缝洞型油藏中水力裂缝扩展规律。首先，通过比较裂缝开度在FEMM和ABAQUS中的计算结果，验证FEMM数值计算结果的有效性；然后，通过模拟带空洞平板中裂缝扩展路径，将扩展路径与相关文献的模拟结果进行比较，结果具有较好的一致性；最后，依次探究单向围压和注水速度对缝洞型油藏中水力裂缝扩展路径的影响规律，并模拟了真三维情况下裂缝扩展规律。文章得到的主要结论如下：

(1) 在缝洞型油藏中，溶洞的存在会显著改变裂缝的扩展路径。水力裂缝在溶洞的影响下，裂缝附近的应力场和位移场均受到干扰，从而导致了裂缝端部的扩展方向改变。

(2) 单向围压对缝洞型油藏中裂缝的偏转路径有显著的影响。当施加较大的单向围压时，裂缝向溶洞方向偏转的趋势较弱；而当减少围压时，水力裂缝向溶洞方向偏转的趋势增强，此时裂缝更容易与溶洞连接，从而更有利于提高石油的开采量。

(3) 注水流速对缝洞型油藏中裂缝偏转路径有显著的影响。当注水流速较大时，裂缝向溶洞方向偏转的趋势较弱；而当注水流速较小时，裂缝偏向溶洞扩展的趋势较强，此时裂缝更容易与溶洞连接，从而更有利于石油开采。

责任编辑：范二平

图 1 被裂缝平面穿过的四面体

Figure 1. Tetrahedral element passed by a planar fracture

下载: 全尺寸图片幻灯片

图 2 网格覆盖域中裂缝单元、桥单元和普通单元示意图

Figure 2. Schematic of the FE, bridge, fracture elements

下载: 全尺寸图片幻灯片

图 3 裂缝单元流体流动路径

Figure 3. Flow path associated with a fracture-element

下载: 全尺寸图片幻灯片

图 4 裂缝单元子区域体积定义

Figure 4. The volume of node associated with a fracture-element

下载: 全尺寸图片幻灯片

图 5 FEMM与Fracflow耦合原理示意图

Figure 5. Scheme of coupling principle between FEMM and Fracflow

下载: 全尺寸图片幻灯片

图 6 裂缝开度验证模型几何示意图

Figure 6. A cubic rock with a central fracture

下载: 全尺寸图片幻灯片

图 7 裂缝开度验证模拟结果

Figure 7. Simulation results of facture aperture

下载: 全尺寸图片幻灯片

图 8 带空洞平板几何图

Figure 8. Geometry of a thin plate with a hole and an edge fracture

下载: 全尺寸图片幻灯片

图 9 带空洞平板裂纹偏转示意图

Figure 9. Fracture propagation near a hole without fluid

下载: 全尺寸图片幻灯片

图 10 裂缝沿y方向扩展位移比较

Figure 10. Comparison of the calculated path along the y direction for the fracture propagation near a hole without fluid with that of the reference

下载: 全尺寸图片幻灯片

图 11 带溶洞立方体几何图

Figure 11. A cubic domain with a cavity and a central fracture

下载: 全尺寸图片幻灯片

图 12 岩体位移场分布

Figure 12. Vertical displacement distribution of the reservoir for hydraulic fracture propagation

下载: 全尺寸图片幻灯片

图 13 不同围压下岩体竖向位移图及裂缝沿y方向的偏转位移比较

Figure 13. Comparison of vertical displacement of the reservoir and fracture propagation path along the y direction under different confining pressures

下载: 全尺寸图片幻灯片

图 14 不同注水速度下岩体竖向位移场分布

Figure 14. Vertical displacement distribution of the reservoir under different water injection velocities

下载: 全尺寸图片幻灯片

图 15 缝洞型油藏三维模型

Figure 15. Geometry of the 3D model of the fractured-cavity reserior

下载: 全尺寸图片幻灯片

图 16 三维缝洞型油藏裂缝偏转示意图

Figure 16. Fracture propagation for the 3D hydraulic fracture propagation near a spherical cavity

下载: 全尺寸图片幻灯片

表 1 裂缝开度验证模型输入参数

Table 1. Input parameters for the cubic rock with a central fracture

输入参数	数值
杨氏模量E/GPa	10
泊松比v	0.25
恒定水压p/kPa	10

下载: 导出CSV

表 2 带溶洞模型输入参数

Table 2. Input parameters for a cubic domain

输入参数	值
杨氏模量E/GPa	9
泊松比v	0.25
抗拉强度f_t/MPa	1
密度ρ/(kg·m^-3)	1100
材料孔隙度	0.1
裂缝孔隙度	0.25
岩体渗透率/(m²·s^-1)	1.0×10^-20
裂缝渗透率/(m²·s^-1)	1.0×10^-10
流体黏度/(Pa·s^-1)	1.0×10^-3
流体注入速率/(kg·s^-1)	6.0×10^-2

下载: 导出CSV

表 3 三维模型输入参数

Table 3. Input parameters for the 3D model

输入参数	值
杨氏模量E/GPa	1
泊松比v	0.20
抗拉强度f_t/MPa	1
密度ρ/(kg·m^-3)	1100
岩体孔隙度	0.06
岩体渗透率/m²	1.0×10^-20
裂缝处渗透率/m²	1.0×10^-10
黏度/(Pa·s^-1)	1.0×10^-3

下载: 导出CSV

参考文献(44)

ADACHI J, SIEBRITS E, PEIRCE A, et al., 2007. Computer simulation of hydraulic fractures[J]. International Journal of Rock Mechanics and Mining Sciences, 44(5):739-757. doi: 10.1016/j.ijrmms.2006.11.006

BELYTSCHKO T, GRACIE R, VENTURA G, 2009. A review of extended/generalized finite element methods for material modeling[J]. Modelling and Simulation in Materials Science and Engineering, 17(4):043001. doi: 10.1088/0965-0393/17/4/043001

FU J W, ZHU W S, ZHANG X Z, et al., 2017. Fracturing experiment and numerical simulation study on new material containing a hollow internal crack under internal water pressure[J]. Advanced Engineering Sciences, 49(4):78-85. (in Chinese with English abstract) http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=scdxxb-gckx201704010

GAO B, HUANG Z Q, YAO J, et al., 2016. Pressure transient analysis of a well penetrating a filled cavity in naturally fractured carbonate reservoirs[J]. Journal of Petroleum Science and Engineering, 145:392-403. doi: 10.1016/j.petrol.2016.05.037

GONG D G, QU Z Q, LI J X, et al., 2016. Extended finite element simulation of hydraulic fracture based on ABAQUS platform[J]. Rock and Soil Mechanics, 37(5):1512-1520. (in Chinese with English abstract) http://d.old.wanfangdata.com.cn/Periodical/ytlx201605036

HAO Z Y, YUE L X, 2018. Thermo-fluid-solid coupling model and numerical simulation of supercritical CO₂ antireflection coal[J]. Advanced Engineering Sciences, 50(4):228-236. (in Chinese with English abstract) http://d.old.wanfangdata.com.cn/Periodical/scdxxb-gckx201804030

KHVATOVA I E, RENAUD A, MALYUTINA G, et al., 2012. Simulation of complex carbonate field: double media vs. single media Kharyaga field case (Russian)[R]. Moscow: Society of Petroleum Engineers.

LIU G W, LI Q B, LIANG G H, 2017. A phase-field description of dynamic hydraulic fracturing[J]. Chinese Journal of Rock Mechanics and Engineering, 36(6):1400-1412. (in Chinese with English abstract) doi: 10.13722/j.cnki.jrme.2016.1075

LIU Q S, SUN L, TANG X H, et al., 2018. Simulate intersecting 3D hydraulic cracks using a hybrid "FE-Meshfree" method[J]. Engineering Analysis with Boundary Elements, 91:24-43. doi: 10.1016/j.enganabound.2018.03.005

MELENK J M, BABUŠKA I, 1996. The partition of unity finite element method:basic theory and applications[J]. Computer Methods in Applied Mechanics and Engineering, 139(1-4):289-314. doi: 10.1016/S0045-7825(96)01087-0

RAJENDRAN S, ZHANG B R, 2007. A "FE-Meshfree" QUAD4 element based on partition of unity[J]. Computer Methods in Applied Mechanics and Engineering, 197(1-4):128-147. doi: 10.1016/j.cma.2007.07.010

RAJENDRAN S, ZHANG B R, 2008. Corrigendum to "A 'FE-Meshfree' QUAD4 element based on partition of unity"[J]. Computer Methods in Applied Mechanics and Engineering, 197(13-16):1430. doi: 10.1016/j.cma.2007.11.012

SHI G H, 1991. Manifold method of material analysis[C]//Proceedings of the transactions of the ninth army conference on applied mathematics and computing. Minnesoda: U.S. Army Research Office: 57-76.

STROUBOULIS T, BABUŠKA I, COPPS K, 2000. The design and analysis of the generalized finite element method[J]. Computer Methods in Applied Mechanics and Engineering, 181(1-3):43-69. doi: 10.1016/S0045-7825(99)00072-9

TANG X H, ZHENG C, WU S C, et al., 2009. A novel four-node quadrilateral element with continuous nodal stress[J]. Applied Mathematics and Mechanics, 30(12):1519-1532. doi: 10.1007/s10483-009-1204-1

WANG L, YANG S L, LIU Y C, et al., 2017. Experiments on gas supply capability of commingled production in a fracture-cavity carbonate gas reservoir[J]. Petroleum Exploration and Development, 44(5):779-787. (in Chinese with English abstract) http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=syktykf201705013

WANG X, ZHU Z M, WANG X M, et al., 2017. Effect of integral paths on the accuracy of finite difference method[J]. Advanced Engineering Sciences, 49(S2):141-149. (in Chinese with English abstract) http://en.cnki.com.cn/Article_en/CJFDTotal-SCLH2017S2020.htm

WITHERSPOON P A, WANG J S Y, IWAI K, et al., 1980. Validity of cubic law for fluid flow in a deformable rock fracture[J]. Water Resources Research, 16(6):1016-1024. doi: 10.1029/WR016i006p01016

WU P F, 2017. Experimental investigation on the crack propagation of hydraulic fracturing in coal-rock combination[D]. Shanxi: Taiyuan University of Technology: 1-50. (in Chinese)

WU Y, DAI J S, GU Y C, et al., 2014. Numerical simulation of present geo-stress field and its effect on hydraulic fracturing of Fuyu reservoir in Gaotaizi oilfield[J]. Journal of Geomechanics, 20(4):363-371. (in Chinese with English abstract) http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=dzlxxb201404004

XIE J, ZHU Z M, HU R, 2015. Propagation criterion and application of sandstone reservoir fractures under hydraulic fracturing[J]. Journal of Sichuan University (Engineering Science Edition), 47(5):38-45. (in Chinese with English abstract) http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=scdxxb-gckx201505006

YAN C Z, ZHENG H, SUN G H, 2016. Effect of in-situ stress on hydraulic fracturing based on FDEM-Flow[J]. Rock and Soil Mechanics, 37(1):237-246. (in Chinese with English abstract) http://d.old.wanfangdata.com.cn/Periodical/ytlx201601028

YANG X, ZHANG G Q, LIU Z B, et al., 2017. Experimental research on the variation of fracture width in hydraulic fracturing process[J]. Chinese Journal of Rock Mechanics and Engineering, 36(9):2232-2237. (in Chinese with English abstract)

YANG Y T, TANG X H, ZHENG H, 2014. A three-node triangular element with continuous nodal stress[J]. Computers & Structures, 141:46-58. doi: 10.1016/j.compstruc.2014.05.001

YANG Y T, ZHENG H, 2016. A three-node triangular element fitted to numerical manifold method with continuous nodal stress for crack analysis[J]. Engineering Fracture Mechanics, 162:51-75. doi: 10.1016/j.engfracmech.2016.05.007

YAO C, ZHAO M, YANG J H, et al., 2017. Improved method of rigid body spring for 2D hydraulic fracturing simulation[J]. Chinese Journal of Rock Mechanics and Engineering, 36(9):2169-2176. (in Chinese with English abstract) http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=yslxygcxb201709009

ZHAO K Z, ZHANG L J, ZHENG D M, et al., 2015. A reserve calculation method for fracture-cavity carbonate reservoirs in Tarim Basin, NW China[J]. Petroleum Exploration and Development, 42(2):277-282. doi: 10.1016/S1876-3804(15)30017-3

ZHAO Z J, LIU D A, CUI Z D, et al., 2019. Cyclic progressive pressure on the fracturing effect of shale[J]. Chinese Journal of Rock Mechanics and Engineering, 38(S1):2779-2789. (in Chinese with English abstract)

ZIENKIEWICZ O C, TAYLOR R L, 2000. The finite element method[M]. 5th ed. Oxford, Boston:Butterworth-Heinemann.

ZU K W, CHENG X S, LUO Z L, et al., 2018. The comparative analysis of different methods for fracture prediction in complex carbonate rock reservoir[J]. Journal of Geomechanics, 24(4):465-473. (in Chinese with English abstract) http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=10.1177/0148607102026001011

付金伟, 朱维申, 张新中, 等, 2017.内水压下含中空裂隙新型材料的压裂试验及数值模拟研究[J].工程科学与技术, 49(4):78-85. http://d.old.wanfangdata.com.cn/Periodical/scdxxb-gckx201704010

龚迪光, 曲占庆, 李建雄, 等, 2016.基于ABAQUS平台的水力裂缝扩展有限元模拟研究[J].岩土力学, 37(5):1512-1520. http://d.old.wanfangdata.com.cn/Periodical/ytlx201605036

郝志勇, 岳立新, 2018.超临界CO₂增透煤热流固耦合模型与数值模拟[J].工程科学与技术, 50(4):228-236. http://d.old.wanfangdata.com.cn/Periodical/scdxxb-gckx201804030

刘国威, 李庆斌, 梁国贺, 2017.动力水力压裂的相场模拟方法[J].岩石力学与工程学报, 36(6):1400-1412. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=yslxygcxb201706009

王璐, 杨胜来, 刘义成, 等, 2017.缝洞型碳酸盐岩气藏多层合采供气能力实验[J].石油勘探与开发, 44(5):779-787. http://d.old.wanfangdata.com.cn/Periodical/syktykf201705013

王雄, 朱哲明, 汪小梦, 等, 2017.不同积分路径对动态有限差分法计算精度的影响效应[J].工程科学与技术, 49(S2):141-149. http://www.cnki.com.cn/Article/CJFDTotal-SCLH2017S2020.htm

武鹏飞, 2017.煤岩复合体水压致裂裂纹扩展规律试验研究[D].山西: 太原理工大学: 1-50.

伍亚, 戴俊生, 顾玉超, 等, 2014.高台子油田扶余油层现今地应力数值模拟及对水力压裂的影响[J].地质力学学报, 20(4):363-371. doi: 10.3969/j.issn.1006-6616.2014.04.004

谢军, 朱哲明, 胡荣, 2015.砂岩储层裂缝在水力压裂作用下扩展准则及其应用[J].四川大学学报(工程科学版), 47(5):38-45. http://d.old.wanfangdata.com.cn/Periodical/scdxxb-gckx201505006

严成增, 郑宏, 孙冠华, 等, 2016.基于FDEM-Flow研究地应力对水力压裂的影响[J].岩土力学, 37(1):237-246. http://d.old.wanfangdata.com.cn/Periodical/ytlx201601028

杨潇, 张广清, 刘志斌, 等, 2017.压裂过程中水力裂缝动态宽度实验研究[J].岩石力学与工程学报, 36(9):2232-2237. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=yslxygcxb201709016

姚池, 赵明, 杨建华, 等, 2017.基于改进刚体弹簧方法的二维水压致裂模型[J].岩石力学与工程学报, 36(9):2169-2176. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=yslxygcxb201709009

赵子江, 刘大安, 崔振东, 等, 2019.循环渐进升压对页岩压裂效果的影响[J].岩石力学与工程学报, 38(S1):2779-2789. http://www.cqvip.com/QK/96026X/2019A01/89837688504849578349484956.html

祖克威, 程秀申, 罗周亮, 等, 2018.复杂碳酸盐岩储层裂缝预测方法对比性研究[J].地质力学学报, 24(4):465-473. http://journal.geomech.ac.cn/ch/reader/view_abstract.aspx?flag=1&file_no=20180403&journal_id=dzlxxb

被引

资源附件(0)

访问统计

图(16) / 表(3)

计量

文章访问数: 363
HTML全文浏览量: 123
PDF下载量: 17
被引次数: 0

0. 引言
1. FEMM-Fracflow基本理论
1.1 FEMM基本理论
1.2 Fracflow基本理论
1.3 FEMM与Fracflow耦合
2. 数值算例
2.1 裂缝开度计算验证
2.2 裂缝扩展路径验证
2.3 溶洞对裂缝扩展的影响研究
2.4 围压对缝洞型油藏中裂缝扩展路径影响
2.5 注水速度对缝洞型油藏中裂缝扩展路径影响
2.6 真三维球体溶洞对裂缝扩展路径的影响
3. 结论

0. 引言
1. FEMM-Fracflow基本理论
1.1 FEMM基本理论
1.2 Fracflow基本理论
1.3 FEMM与Fracflow耦合
2. 数值算例
2.1 裂缝开度计算验证
2.2 裂缝扩展路径验证
2.3 溶洞对裂缝扩展的影响研究
2.4 围压对缝洞型油藏中裂缝扩展路径影响
2.5 注水速度对缝洞型油藏中裂缝扩展路径影响
2.6 真三维球体溶洞对裂缝扩展路径的影响
3. 结论

参考文献(44)

被引

资源附件(0)

访问统计

姓名
邮箱
手机号码
标题
留言内容
验证码

留言板

基于FEMM-Fracflow研究缝洞型油藏中裂缝扩展问题

doi: 10.12090/j.issn.1006-6616.2020.26.01.006

作者简介:
王慧(1994-), 女, 在读硕士, 研究方向为多物理场裂缝扩展问题。E-mail:huiwangwhu@163.com

通讯作者:
刘泉声(1962-), 男, 教授, 从事岩石力学方面研究。E-mail:2992464906@qq.com

计量

Investigation on fracture propagation in fractured-cavity reservoirs based on FEMM-fracflow modelling

0. 引言

1. FEMM-Fracflow基本理论

1.1 FEMM基本理论

1.1.1 单位分解函数的形成

1.1.2 裂缝单元近似函数构造

1.1.3 岩体本构关系及裂缝扩展条件

1.2 Fracflow基本理论

1.2.1 流体计算控制方程

1.2.2 流体压力的更新

1.3 FEMM与Fracflow耦合

2. 数值算例

2.1 裂缝开度计算验证

2.2 裂缝扩展路径验证

2.3 溶洞对裂缝扩展的影响研究

2.4 围压对缝洞型油藏中裂缝扩展路径影响

2.5 注水速度对缝洞型油藏中裂缝扩展路径影响

2.6 真三维球体溶洞对裂缝扩展路径的影响

3. 结论

计量

目录

0. 引言

1. FEMM-Fracflow基本理论

1.1 FEMM基本理论

1.2 Fracflow基本理论

1.3 FEMM与Fracflow耦合

2. 数值算例

2.1 裂缝开度计算验证

2.2 裂缝扩展路径验证

2.3 溶洞对裂缝扩展的影响研究

2.4 围压对缝洞型油藏中裂缝扩展路径影响

2.5 注水速度对缝洞型油藏中裂缝扩展路径影响

2.6 真三维球体溶洞对裂缝扩展路径的影响

3. 结论

留言板

基于FEMM-Fracflow研究缝洞型油藏中裂缝扩展问题

doi: 10.12090/j.issn.1006-6616.2020.26.01.006

作者简介: 王慧(1994-), 女, 在读硕士, 研究方向为多物理场裂缝扩展问题。E-mail:huiwangwhu@163.com

通讯作者: 刘泉声(1962-), 男, 教授, 从事岩石力学方面研究。E-mail:2992464906@qq.com

计量

出版历程

Investigation on fracture propagation in fractured-cavity reservoirs based on FEMM-fracflow modelling

0. 引言

1. FEMM-Fracflow基本理论

1.1 FEMM基本理论

1.1.1 单位分解函数的形成

1.1.2 裂缝单元近似函数构造

1.1.3 岩体本构关系及裂缝扩展条件

1.2 Fracflow基本理论

1.2.1 流体计算控制方程

1.2.2 流体压力的更新

1.3 FEMM与Fracflow耦合

2. 数值算例

2.1 裂缝开度计算验证

2.2 裂缝扩展路径验证

2.3 溶洞对裂缝扩展的影响研究

2.4 围压对缝洞型油藏中裂缝扩展路径影响

2.5 注水速度对缝洞型油藏中裂缝扩展路径影响

2.6 真三维球体溶洞对裂缝扩展路径的影响

3. 结论

计量

出版历程

目录

0. 引言

1. FEMM-Fracflow基本理论

1.1 FEMM基本理论

1.2 Fracflow基本理论

1.3 FEMM与Fracflow耦合

2. 数值算例

2.1 裂缝开度计算验证

2.2 裂缝扩展路径验证

2.3 溶洞对裂缝扩展的影响研究

2.4 围压对缝洞型油藏中裂缝扩展路径影响

2.5 注水速度对缝洞型油藏中裂缝扩展路径影响

2.6 真三维球体溶洞对裂缝扩展路径的影响

3. 结论

作者简介:
王慧(1994-), 女, 在读硕士, 研究方向为多物理场裂缝扩展问题。E-mail:huiwangwhu@163.com

通讯作者:
刘泉声(1962-), 男, 教授, 从事岩石力学方面研究。E-mail:2992464906@qq.com