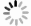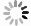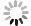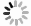LIMIT ANALYSIS OF PERMANENT DISPLACEMENT FOR SLOPE CONSIDERING THE TENSILE STRENGTH OF SOIL
-
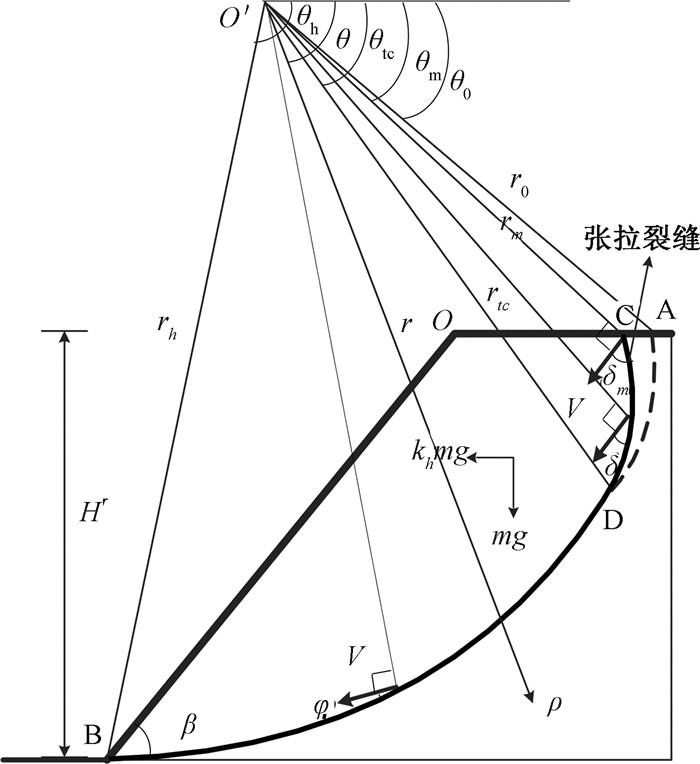
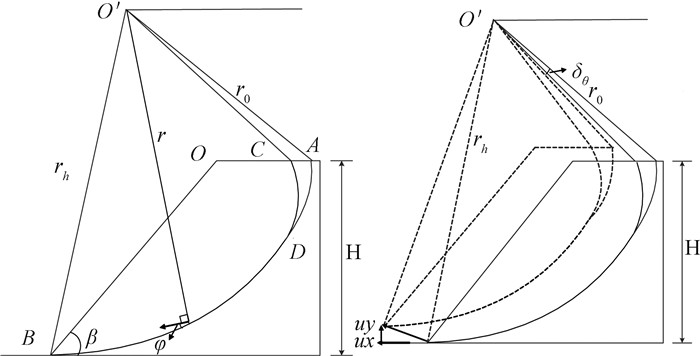
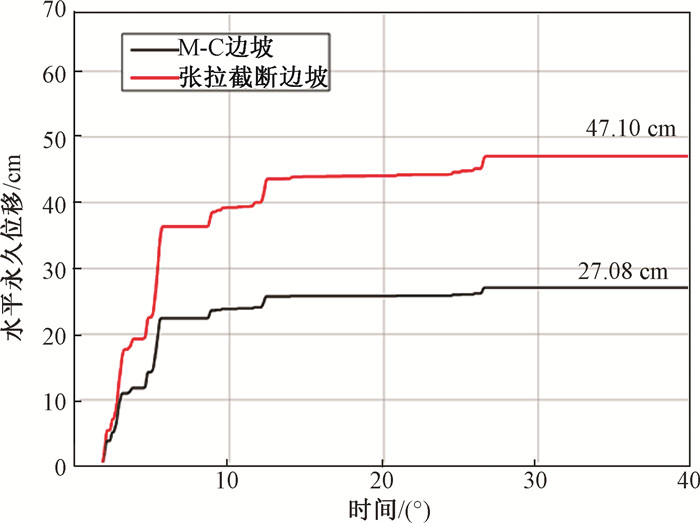
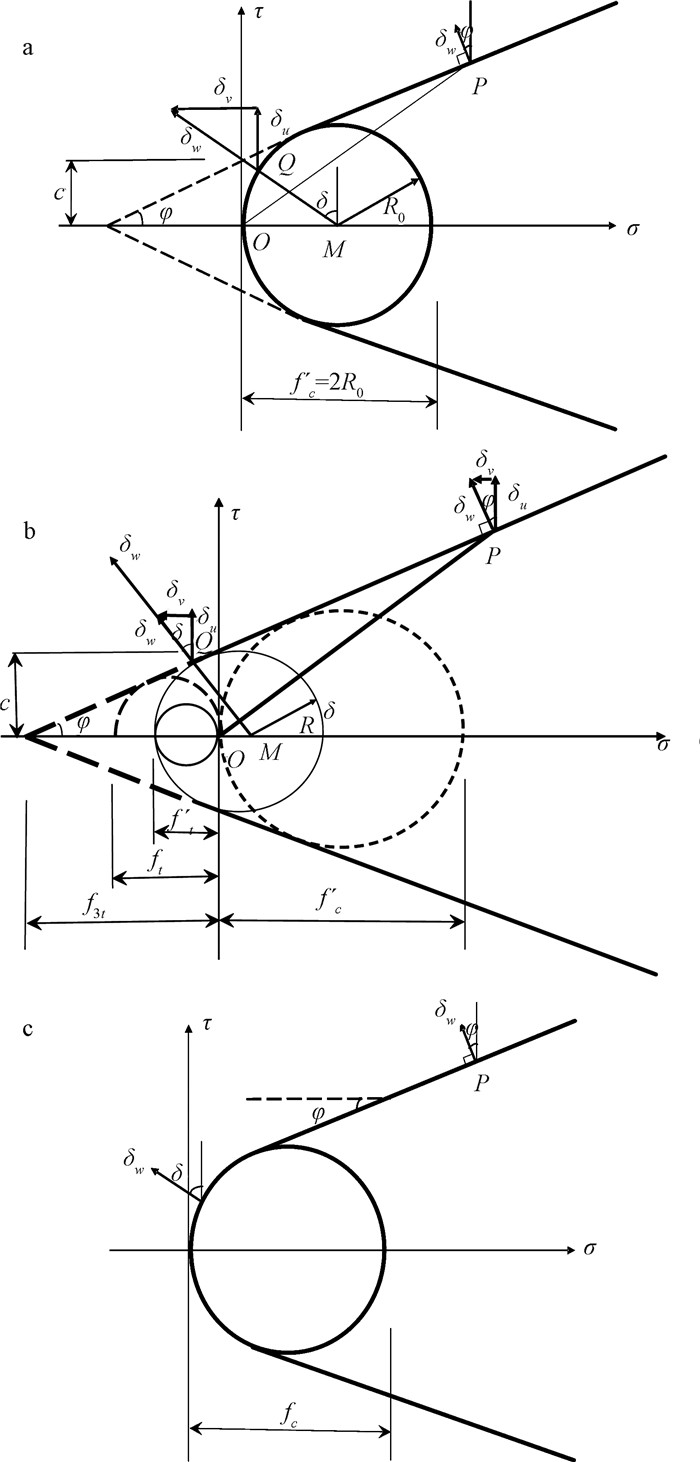
摘要: 通过野外观测与室内试验发现,边坡后缘往往存在拉应力区。拉应力区的存在会影响边坡的稳定性,而地震荷载的存在会放大这种影响。分析拉应力区对边坡稳定性的影响,当前主要采用的方式为:对强度准则中抗拉强度进行折减(即张拉截断)。文章通过极限分析上限原理和拟静力法,推导出边坡临界加速度计算方程。以边坡在不同参数组合下的位移系数为基础,输入实测地震波,采用改进的Newmark法对边坡进行位移分析。文章算例的结果表明:拉应力区的存在会大大降低边坡临界加速度,土体在完全张拉截断下的临界加速度对边坡可能会产生超过50%的折减。拉应力区的存在也可以使永久位移达到传统的摩尔库伦理论计算值的2倍之多。文中所有的结果皆以图表形式展示,非常便于理解以及读取数据。Abstract: Through field observation and laboratory experiment, it is found that the stability of the slope is influenced by the existence of tensile stress zone in the back edge of the slope, while the influence is amplified by the existence of seismic load. To analyze the impact of tensile stress zone on the stability of slope, the main method used at present is to reduce the tensile strength in the strength criterion (i.e., tension cut-off). According to the upper limit principle of limit analysis and the quasi-static method, the calculation equation of the critical acceleration of the slope is derived. Based on the displacement coefficients of the slope under different parameter combinations, the measured seismic wave was input and the improved Newmark method was used to analyze the displacement of the slope. The results show that the critical acceleration of the slope can be greatly reduced by the existence of the tensile stress area, and the critical acceleration of the soil mass under the complete tension cut-off may produce more than 50% reduction of the slope. The existence of the tensile stress zone can also make the permanent displacement as much as twice the value calculated by the traditional Mohr-Coulomb yield criterion. All of the results in this article are presented in graphical form, which is very easy to understand and read.
-
Key words:
- seismic load /
- limit analysis /
- tension cut-off /
- critical acceleration /
- permanent displacement
-
胶东西北部金矿集中区作为我国黄金资源和生产的重要基地, 以其得天独厚的成矿地质条件和丰富的金矿资源享誉世界, 引起了中外众多地学工作者的瞩目。近几十年来, 该区投入了大量地质科研工作, 内容涉及多个地学领域。然而大多数研究集中在单一构造或构造组合对矿化的控制上, 很少考虑构造在时空上的演化。
众所周知, 矿床或矿带是地球有用物质高度富集的结果, 是地球演化过程中特定条件下的产物[1], 而成矿作用过程更是一个复杂的、开放的、非线性的作用系统[2], 一个矿床的形成既与历史时期的区域成矿背景和特定的成矿动力学条件有关, 也与成矿后期的变化与保存有关[1]。因此本文试图以胶东西北部金成矿带为例, 以系统分析的思想和方法探讨矿化形成的过程, 把成矿作用与区域地质演化有机地联系起来, 全面、历史地研究区域矿化特征和构造控矿规律。
1. 胶东西北部金成矿带构造分布特征
胶西北金成矿带地处胶东半岛西部, 其西侧与郯庐断裂带毗邻, 是中-新生代大陆边缘裂谷构造-岩浆和成矿作用频繁活动的地带[3]。该区的构造格局主要是在古生代构造格架基础上发展演变而来的, 以大规模的构造岩浆作用为主[4]。
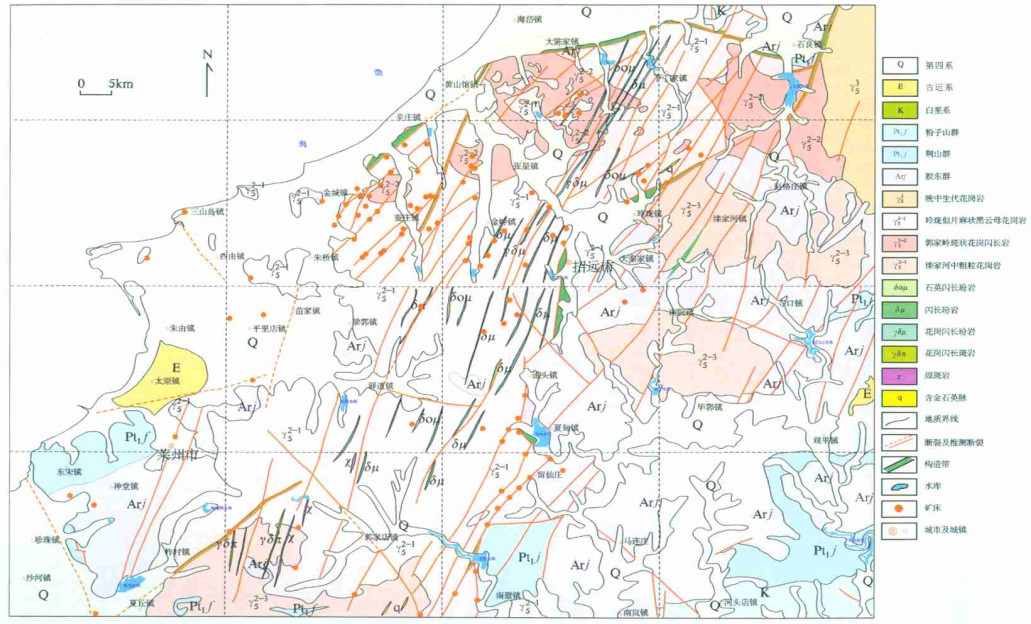
胶西北成矿带主要受基底东西向栖霞复背斜构造及北东向断裂构造系统共同控制。栖霞复背斜控制金矿带总体的东西展布, 而北东向断裂则控制了金矿化的产出空间(图 1)。
栖霞复背斜是该区最主要的东西向基底构造。该复背斜由前寒武纪胶东群和粉子山群组成, 总体上近东西向展布, 背斜中段即为胶东西北部的金矿成矿带。
1 栖霞复背斜
2 北东、北北东向断裂构造带
从西到东主要有三山岛断裂带、焦家断裂带和招平断裂带。
三山岛断裂带分布于濒临渤海的三山岛, 沿胶东群和玲珑岩体接触带产出。断裂走向北东40°, 倾向南东, 倾角30~40°, 平面上呈舒缓波状, 矿床(点)均赋存于断裂面下伏的构造蚀变破碎岩区。焦家断裂带由主干断裂及其派生的分支断裂组成, 走向大约北东30°, 倾向北西, 倾角30~45°, 断裂具有连续稳定的主断面和一套发育完整的构造蚀变带。
招平断裂带南起平度市, 向北延至招远以北, 总体呈"S"型展布[5]。关于该断裂的组成及空间展布一直是地学工作者关注的焦点[3~4]。
2. 胶东西北部金成矿带构造形变特征
三山岛、焦家和招平成矿带是多次活动的复杂构造带[5~8]。早期韧性剪切作用发生在早先存在的断层软弱带或岩性界面上, 形成各种糜棱岩。晚期脆性变形叠加在早期韧性变形上, 形成各种类型的碎裂构造岩。根据其结构构造特征, 可划分为糜棱岩系列和碎裂岩系列。糜棱岩系列主要沿剪切构造带发育, 受晚期脆性活动的影响, 延续较差, 保存得亦不完善, 可划分为糜棱岩化花岗岩、初糜棱岩、糜棱岩及超糜棱岩, 矿物组合主要为石英、绢云母、斜长石和绿泥石等, 韧性构造如糜棱片理和拔丝构造常见。碎裂岩系列为断裂晚期活动的产物, 沿剪切带中心由近至远依次为碎粉岩、断层泥、碎粒岩、碎斑岩、碎裂岩及碎裂花岗岩。
以招平断裂带为例, 该带在成生演化过程中留下了丰富多彩的岩石类型(图 2)。概括有:各种糜棱岩、糜棱岩化花岗岩、长英质构造角砾岩、糜棱质复构造岩、长英质碎斑岩、碎粒岩、碎裂花岗岩、碎裂变质岩及断层泥等。上述构造动力变质岩大都发育在招平断裂西侧(下盘)玲珑花岗岩中。平面分带总的趋势由主断面向西(或NW)边的玲珑花岗岩一侧, 依次出现糜棱岩、长英质碎斑岩、碎粒岩、构造角砾岩及碎裂花岗岩等; 主断面向东(上盘)边的胶东变质岩一侧, 依次出现泥化糜棱岩、泥化碎裂变质岩等, 沿主断面有一层比较稳定的断层泥。由此可见, 成岩作用在剖面上并不具对称分带性, 在岩体内更发育, 其原因与上下盘的岩石力学性质有关。
3. 控矿构造系统分析
所谓控矿构造系统分析就是把成矿作用置于地壳物质循环当中, 从时间、空间上解析成矿作用与地质构造事件的成生联系, 从历史角度去考察不同地质构造事件之间的相关关系及对成矿的贡献大小, 再现不同历史时期的成矿过程。
3.1 深部构造对金矿化的控制
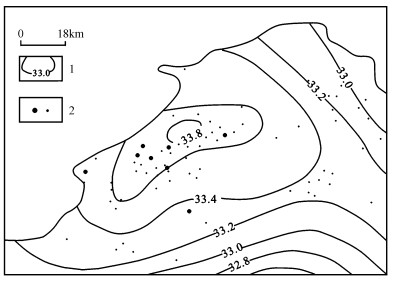
深部构造活动对一个地区的构造-岩浆活动有着重要的影响。前人曾对该区的深部构造进行了详细研究[4, 9~10], 认为在该区深部存在一明显的东西向构造带, 主要由重熔的玲珑片麻状花岗岩及郭家岭二长花岗岩组成, 该区多具有幔源性质的中基性脉岩中的花岗岩捕虏体也旁证了这一点[10]。这些暗绿色的基性脉岩, 其成岩年龄大多集中在80~100Ma之间[6], 可能为中新生代深部活动的产物, 初步推测与地幔柱的上升有关。毛景文等[11]对胶东西北部几个典型金矿床的碳氢氧同位素测定结果表明, 在120Ma左右整个胶东, 乃至整个华北克拉通区发生了岩石圈减薄、软流圈上涌, 这一时期不仅使大量地壳重熔成花岗质岩浆, 还伴随着大规模的深变质作用和地幔脱气。在深大断裂附近, 尤其是花岗岩体为主岩时, 成矿物质有更多的地幔源烙印。最新的区域莫霍面等深资料表明(图 3), 本区深部为区域北东向与近东西向梯度带的交会部位, 也是莫霍面的起伏变化部位, 几个矿化蚀变带恰好位于地幔深部变化的变异带上。地幔的梯度变化带是地壳的薄弱带, 是深部构造的反映, 梯度越大, 各种物理和化学条件的变化也越强烈, 同时该带也是一个高渗透带, 有利于构造岩浆活动的发生。类似的情况在我国其他金矿化区如冀东、小秦岭都有发现[12~13]。
因此可以看出, 在古老的胶东群矿源层的基础上, 由于受深部地幔柱上隆的影响, 胶西北矿化集中区在过去地壳演化的过程中发生过强烈的岩浆活动, 上隆的地幔柱不仅为金矿提供了部分成矿物质[14], 而且在浅部引起强烈的构造活动及变质作用, 从而为矿床的形成提供了有利的热动力条件, 使金矿床与胶东群、重熔花岗岩之间具有同源演化的特征[5, 15~16]。
3.2 断裂构造对金矿化的多级多层次控制
断裂构造对本区金矿的多级多层次控制作用较为明显。金矿化的空间分布, 大至成矿区带, 小至矿体乃至矿石结构构造, 无不受构造特别是断裂的控制, 并且明显表现出多级有序的特点。首先深部构造控制了胶西北矿集区或者成矿带的形成及展布; 其次北东向的断裂构造控制了重熔花岗岩的形成, 进而控制了矿床(点)的空间分布, 而次级断裂裂隙则控制了矿体的产出特征及矿化类型的差异。
例如在焦家金矿田, 焦家断裂及其伴生、派生的次级断裂系统控制了焦家矿田整个的金矿化, 成生在主构造带中的焦家、新城等矿床多为蚀变岩型, 矿床规模大, 矿体较厚且空间延伸稳定; 而其次级断裂如河东-望儿山断裂、河西断裂等则控制了具体中小型矿床的产出, 由于其与主断裂一样经历了多期构造-热液活动, 具有相似的构造环境, 因此控矿部位与前者有较好的对应关系, 但矿化蚀变强度、矿床规模均较主断裂中的矿床弱或小, 多以蚀变岩-石英脉混合型为主, 矿体厚度小且空间变化较大。其他更次级的断裂以及与其伴生的节理裂隙密集带一起控制了小型矿床、矿体的形成、分布、形态和矿石类型(表 1)。
表 1 构造对金矿化的多级多层次控制Table 1. Structural controls on stacking and multi-level gold mineralization
3.3 构造变形岩相对金矿化的控制
在构造应力作用下, 处于不同构造带的岩石往往产生物理性质和岩石学上的变异, 而这种变异直接或间接地控制着成矿作用过程中的物理化学参量。因此即使在相同或相似的地质条件下, 局部变形性质不同的构造地域也具有差异的构造物理化学环境(表 2)。
表 2 构造变形岩相形迹的构造物理化学综合参量特征Table 2. Characteristics of tectono-physico-chemical parameters of tectonic deformation facies
研究发现[5~6], 玲珑型花岗岩是在相对中等压力、还原条件和较高温度下, 郭家岭型花岗闪长岩是在相对高压、较强还原条件和较低温度下, 而滦家河型花岗岩是在较低压力、偏氧化条件和较高温度的相对开放条件下形成的, 并且按照剪切-挤压-引张岩相的顺序逐次成岩的, 磁组构研究也证实了这一特征[17]。因此, 从构造变形花岗岩相对金矿的控制来看, 主要大型金矿产于玲珑型花岗岩内部及边部地带; 而一些中小型金矿床分布在郭家岭型花岗闪长岩与玲珑型花岗岩的接触带, 内部也有分布; 但滦家河型岩体则主要在与围岩的接触带, 特别是在0~5km的外接触带范围内有金矿分布。
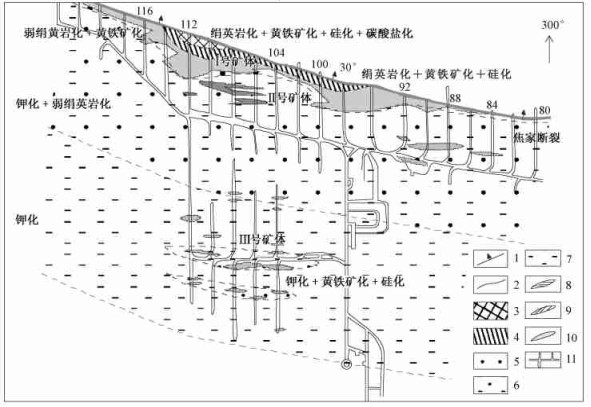
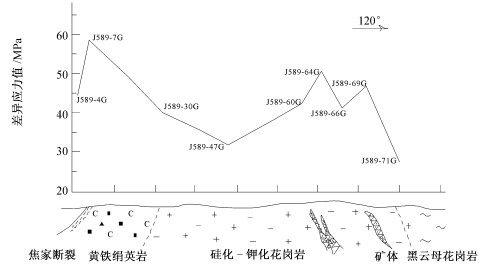
而对于同一构造变形花岗岩相带不同构造部位的3个蚀变带来说[3, 5~7, 15~19], 由于所处温度、压力、应力及应变的不同, 因而出现不同的矿化类型。在主断面附近, 由于温度压力较高, 岩石应力应变强烈, 岩石破碎, 容矿裂隙细小, 所以易形成浸染状蚀变岩型金矿化, 如焦家金矿的Ⅰ号矿脉、新城金矿的主矿体; 而在远离主断面的低级序节理裂隙中, 由于应力应变的空间不均一性及作用范围的影响, 岩石机械破碎较弱, 构造岩强度低, 反映的是一种低温低压的高速应变, 所以大多以细脉(网脉)状矿化为主, 如焦家金矿的Ⅲ号矿脉、望儿山金矿体; 在两者之间多出现过渡类型的矿化, 如焦家金矿的Ⅱ号脉体。由此可见, 不同的构造变形岩相环境往往控制着不同类型的矿化(图 4)。笔者等对焦家金矿1:1000的构造变形岩相形迹实测填图及应力分析发现, 从焦家断裂下盘压剪构造带向南东方向张剪性构造带, 构造蚀变强度逐渐减弱, 矿床由黄铁绢英岩型逐渐转变为黄铁矿石英脉型(图 5), 反映了构造控制矿床类型的这种稳定关系, 即构造带的不同构造部位控制金矿的类别分布。尽管这些金矿床或金矿体具有差异的类型及外貌特征, 但是它们都是同一成矿作用的产物, 具有相似的成矿温度、稳定同位素、稀土配分组成等[5~6, 15~16], 其差异仅仅表现在形态、矿化形式及矿石自然类型等方面, 而这些都是由于控岩控矿及成岩成矿构造部位不同、性质的差别而造成的。
3.4 控矿构造的时空结构
成矿作用过程是一个复杂的巨系统, 是地球物质循环过程中特定地质条件环境的产物。因此, 矿化作用的发生有其内在的作用区域和时间范畴。本文仅讨论区域尺度条件下成矿控矿系统的空间结构和时间结构, 借以探索胶西北金成矿带的矿化分布规律。
3.4.1 控矿构造系统的空间结构
(1) 断裂构造控制金矿化的丛聚性分布
从区域上来说, 胶西北金成矿带中已经发现了不同规模的矿床多达数百个, 主要分布在三山岛、焦家和玲珑3个金矿田, 每个矿田内又可划分出一系列北东向的矿化蚀变带, 这些矿化蚀变带中又集中发育了诸多的金矿床(点)。这些金矿化的丛聚性分布严格受断裂构造的分布控制, 断裂构造及其内部系统发育的不均衡性导致矿化的丛聚性分布。
(2) 构造控制金矿化的似等间距分布
似等间距分布是胶西北金矿化空间结构的又一特征, 并且具有级别之分, 可以是不同矿带之间的似等间距产出, 也可以是同一矿带中不同矿脉之间的似等间距产出, 或者是同一矿脉内的不同矿体之间的似等间距产出, 这种特征在区域范围内的各种尺度上都有所表现, 其明显受控于断裂构造的似等间距分布。
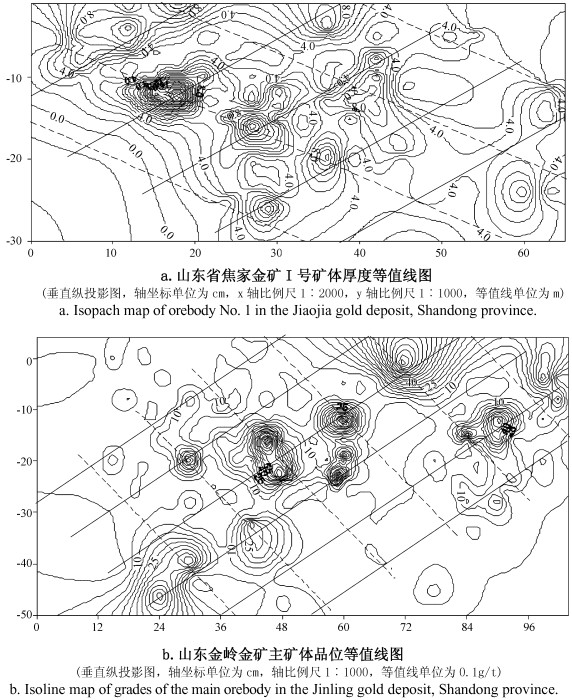
从区域上看, 自西向东三山岛与焦家含矿带的间距为5km, 焦家与玲珑-破头青含矿带的间距为9km, 而破头青与马家窑含矿带的间距为13km, 具有明显的似等间距递变规律; 从同一含矿带上的矿床分布来看, 也具有明显的似等间距分布规律, 如马塘金矿距焦家金矿1.8km, 焦家金矿距东季金矿1.6km, 东季金矿距红布金矿1.5km; 再从矿床内矿体的分布来看, 如图 6所示, 矿化明显受两个侧伏方向的构造所控制, 矿化浓集中心恰好在两组构造趋势线交会处等间距分布。
造成金矿床或矿体似等间距分布的原因, 一方面可能与同一构造体系下形成的不同方向的构造线似等距分布有关, 共轭断裂交会部位往往是成矿的有利部位; 另一方面可能与构造应力波的周期性传递有关。王仁等[20]对此现象进行力学分析后指出, 由于断裂的发生, 某个应力分量减小了, 在断裂附近的一定范围内应变能量得到某种程度的释放, 如果外力不变, 则在这个范围内就不再会发生同一性质的断裂, 并由此导致了断裂构造的等距发育。刘石年[21]、孙丰月[10]等通过对胶东西北部金矿床的研究认为, 断裂面常具波状起伏、凹凸变化的特点, 其分布规律符合主波长理论, 构造面的波峰-波谷-波峰变化与矿化间隔-矿体-矿化间隔是相对应的, 断裂两盘在相对错动中形成的引张空间分布常具等距性, 从而使矿床(点)、矿体及矿柱具等距分布的规律。
(3) 断裂构造对金矿床(体)侧伏侧列的控制
矿体侧伏、侧列是构造与成矿流体运移综合作用的产物, 胶东西北部金矿大多存在这种现象。研究表明, 除少数陡倾、以水平剪切为主运动的断裂控制的矿体无明显侧伏外, 多数受断裂控制的矿体均有侧伏, 而且金矿体侧伏方向与断裂产状有着明显的对应关系, 断裂倾向NW时, 矿床向SW侧伏, 如焦家金矿的Ⅰ号矿体, 而断裂倾向SE时, 矿床则向NE侧伏, 如玲南台上金矿床。矿体的侧伏主要受断裂构造两盘相对运动过程中产生的引张空间的侧伏制约, 而引张空间的侧伏与断裂的性质、运动方式有关, 侧伏角的大小取决于运动方向与断裂面总体走向的交角大小。就焦家金矿田来说, 填图结果表明[5~6, 18~19], 以焦家断裂为活动界面带, 两侧岩块沿一个轨迹发生往返的双向运动, 是矿体侧伏侧列分布的原因; 后期在与早期运移轨迹近于直角的方向上, 又发生上下的往返复合叠加构造运动, 从而造成矿床中的富矿体、矿脉在剖面上呈侧列分布。
(4) 断裂构造对金矿化空间分带的控制
对于胶西北金成矿带矿化的空间分带前人已做了大量的工作[5, 7, 22~23], 也提出了不同的认识。笔者等从构造变形地域出发, 认为区域性压扭断裂中赋存有"焦家式"金矿, 而在其下盘的低级序构造中充填有"玲珑式"金矿, 两者在水平方向上有分带和渐变过渡的关系。例如在玲珑金矿田, 从破头青断裂向北西方向(远离主控矿构造带的方向), 矿化类型呈现极其规律的空间变化:蚀变岩型矿化→过渡型矿化→石英脉型矿化。矿物组合也有类似的变化规律:近破头青断裂以黄铁矿和石英为主, 远离破头青断裂则多金属硫化物含量逐渐增多。在焦家金矿田也存在类似的规律。因此, 可以利用矿化类型的水平分带进行成矿预测。
3.4.2 控矿构造的时间结构
成矿作用是一个复杂的、漫长的作用过程, 由于区域地质背景演化和构造作用的多期多阶段性, 必须对控矿构造的作用过程进行动态的分析。
(1) 区域构造的演化
太古宙时, 由于胶东运动的影响, 地壳受到强烈的南北向挤压, 形成复杂的近东西向的栖霞复背斜及相应的东西向断裂, 奠定了本区的构造基础; 中生代燕山期时, 强烈的构造-岩浆作用不仅导致地壳部分重熔形成金矿的直接矿源岩系——三类花岗岩, 而且形成一系列北东、北北东向的压扭性断裂构造, 从而形成了胶西北现今的构造格架。这些新华夏系断裂构造经历过多次的构造活动, 不仅在早期韧性变形的基础上又叠加脆性变形, 而且由于区域构造应力场的转变[5, 10, 24], 使得其成为很好的导矿和容矿空间, 从目前来看绝大多数的金矿都成生于中生代晚期(100~120Ma)[25~27]。
(2) 控矿构造岩带的时空演化
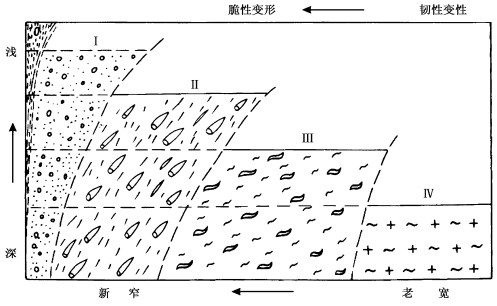
胶西北金矿主要发育在活化地台区含金剪切带中, 因此矿床的形成与剪切岩带的时空演化有着密切的联系。研究表明, 花岗岩成岩期韧性变形的主构造方向为SE-NW或近于EW方向, 而蚀变成矿期的韧性构造以NE、NNE近于SN方向延伸为主要特征[1, 5~8, 26~27]。从胶东地区中生代构造岩带的时空演化模式(图 7)来看, 断裂蚀变岩带从外(似片麻状花岗岩)到内, 有从老→新、从韧性变形→脆性变形、从宽→窄分布, 并从深层→浅层次的复杂多种性质、多期次、多层次的形成特点。同时从发展的观点来看, 由于该区的断裂经历了多次构造活动, 叠加现象明显。其中韧-脆性叠加断裂多发育有大型的蚀变岩型矿床, 如焦家金矿床等; 脆-脆性叠加断裂多成生充填型的石英脉型矿床, 如玲珑金矿等; 而变形及破碎程度介于两者之间的断裂往往形成过渡类型的矿床, 如望儿山金矿床等。再从时间上来看, 从早到晚的矿化过程中, 矿床分布有从岩相内部向边缘相, 进而向外接触带一定范围集中的变化趋势。
(3) 矿化的多期多阶段性
在胶西北地区, 金矿化大致可划分成4个成矿阶段[3, 5, 15~16], 依次为:Ⅰ、黄铁矿-石英阶段, Ⅱ、石英-黄铁矿阶段, Ⅲ、多金属硫化物阶段和Ⅳ、石英-碳酸盐-黄铁矿阶段。其中Ⅱ、Ⅲ成矿阶段是形成工业金矿化的重要阶段, 第Ⅳ阶段虽然仅代表矿化的尾声, 本身也并不形成工业金矿化, 然而对矿化脉的矿化强度具有某种指示意义。
4. 胶西北构造控矿规律
从以上分析可以看出, 胶西北金矿床的产出与构造有着密切的联系。构造运动的发生和发展不仅对胶东地壳演化起着主导作用, 而且对矿床成生具有重要的控制作用, 其具有以下几个特点。
(1) 深部纬向构造控制了胶西北金成矿带的空间展布, 而浅部北东向断裂构造则控制了矿床及矿体的产出。
(2) 断裂构造不仅控制着矿床的产出, 还控制着矿床的规模、矿体的形态、产状及矿床的类型, 具有多层次、多级的特点; 不同矿化类型是同一构造体系下不同构造物理化学环境条件下的产物, 具有同源演化特性。
(3) 断裂构造的空间分布特点控制了矿床的丛聚性、似等间距分布、矿体的侧伏侧列及水平分带, 矿床(点)的分布具有"横向成带"或"行列成阵"的规律, 在空间上具有层次性、有序性和相关性。
(4) 区域构造演化和断裂构造的脆韧性叠加造就了矿化的多样性及复杂性。
-
-
[1] Keefer D K. Statistical analysis of an earthquake-induced landslide distribution-The 1989 Loma Prieta, California event[J]. Engineering Geology, 2000, 58(3~4):231~249. doi: 10.1016/S0013-7952(00)00037-5 [2] Meehan C L, Vahedifard F. Evaluation of simplified methods for predicting earthquake-induced slope displacements in earth dams and embankments[J]. Engineering Geology, 2013, 152(1):180~193. doi: 10.1016/j.enggeo.2012.10.016 [3] Baker R, Shukha R, Operstein V, et al. Stability charts for pseudo-static slope stability analysis[J]. Soil Dynamics and Earthquake Engineering, 2006, 26(9):813~823. doi: 10.1016/j.soildyn.2006.01.023 [4] Zhang K, Cao P. Slope seismic stability analysis on kinematical element method and its application[J]. Soil Dynamics and Earthquake Engineering, 2013, 50:62~71. doi: 10.1016/j.soildyn.2013.03.002 [5] Zhao L H, Cheng X, Zhang Y B, et al. Stability analysis of seismic slopes with cracks[J]. Computers and Geotechnics, 2016, 77:77~90. doi: 10.1016/j.compgeo.2016.04.007 [6] Yang C W, Zhang J J, Fu X, et al. Improvement of pseudo-static method for slope stability analysis[J]. Journal of Mountain Science, 2014, 11(3):625~633. doi: 10.1007/s11629-013-2756-8 [7] Newmark N M. Effects of earthquakes on dams and embankments[J]. Géotechnique, 1965, 15(2):139~160. doi: 10.1680/geot.1965.15.2.139 [8] Rathje E M, Bray J D. Nonlinear coupled seismic sliding analysis of earth structures[J]. Journal of Geotechnical and Geoenvironmental Engineering, 2015, 126(11):1002~1014. http://cn.bing.com/academic/profile?id=c25c5bb2f3233c92363ffd4b9cc9120d&encoded=0&v=paper_preview&mkt=zh-cn [9] You L Z, Michalowski R L. Displacement charts for slopes subjected to seismic loads[J]. Computers and Geotechnics, 1999, 25(1):45~55. doi: 10.1016/S0266-352X(99)00016-6 [10] Utili S, Abd A H. On the stability of fissured slopes subject to seismic action[J]. International Journal for Numerical and Analytical Methods in Geomechanics, 2016, 40(5):785~806. doi: 10.1002/nag.v40.5 [11] Zhao L H, Cheng X, Dan H C, et al. Effect of the vertical earthquake component on permanent seismic displacement of soil slopes based on the nonlinear Mohr-Coulomb failure criterion[J]. Soils and Foundations, 2017, 57(2):237~251. doi: 10.1016/j.sandf.2016.12.002 [12] Zhao L H, Cheng X, Li L, et al. Seismic displacement along a log-spiral failure surface with crack using rock Hoek-Brown failure criterion[J]. Soil Dynamics and Earthquake Engineering, 2017, 99:74~85. doi: 10.1016/j.soildyn.2017.04.019 [13] 尤明庆.均质土坡滑动面的变分法分析[J].岩石力学与工程学报, 2006, 25 http://d.old.wanfangdata.com.cn/Periodical/yslxygcxb2006z1021S1):2735~2745. YOU Mingqing. Study on landslide of homogeneous soil with calculus of variations[J]. Chinese Journal of Rock Mechanics and Engineering, 2006, 25(S1):2735~2745. (in Chinese with English abstract) http://d.old.wanfangdata.com.cn/Periodical/yslxygcxb2006z1021 [14] Duncan J M, Wright S G. Soil strength and slope stability[M]. Hoboken:Wiley, 2005. [15] Utili S. Investigation by limit analysis on the stability of slopes with cracks[J]. Géotechnique, 2013, 63(2):140~154. doi: 10.1680/geot.11.P.068 [16] Michalowski R L. Stability of intact slopes with tensile strength cut-off[J]. Géotechnique, 2017, 67(8):720~727. doi: 10.1680/jgeot.16.P.037 [17] Kaniraj S R, Abdullah H. Effect of berms and tension crack on the stability of embankments on soft soils[J]. Soils and Foundations, 1993, 33(4):99~107. doi: 10.3208/sandf1972.33.4_99 [18] Baker R. Tensile strength, tension cracks, and stability of slopes[J]. Soils and Foundations, 1981, 21(2):1~17. doi: 10.3208/sandf1972.21.2_1 [19] Park D, Wang Z J, Michalowski R L. Consequences of seismic excitation on slopes in soils with a tensile strength cutoff[A]. Geotechnical Frontiers 2017[C]. Orlando, Florida: American Society of Civil Engineers, 2017: 304~313. [20] Taylor D W. Fundamentals of soil mechanics[J]. Soil Science, 1948, 66(2):161. doi: 10.1227-NEU.0b013e31822b8107/ [21] Chen W F. Limit analysis and soil plasticity[M]. Amsterdam:Elsevier, 1975. [22] Chang C J, Chen W F, Yao J T P. Seismic displacements in slopes by limit analysis[J]. Journal of Geotechnical Engineering, 1984. 110(7):860~874. doi: 10.1061/(ASCE)0733-9410(1984)110:7(860) [23] Michalowski R L, You L Z. Displacements of Reinforced Slopes Subjected to Seismic Loads[J]. Journal of Geotechnical and Geoenvironmental Engineering, 2000, 126(8):685~694. doi: 10.1061/(ASCE)1090-0241(2000)126:8(685) [24] Li X P, He S M, Wu Y. Seismic displacement of slopes reinforced with piles[J]. Journal of Geotechnical and Geoenvironmental Engineering, 2010, 136(6):880~884. doi: 10.1061/(ASCE)GT.1943-5606.0000296 [25] Nadukuru S S, Michalowski R L. Three-dimensional displacement analysis of slopes subjected to seismic loads[J]. Canadian Geotechnical Journal, 2013, 50(6):650~661. doi: 10.1139/cgj-2012-0223 [26] He Y, Hazarika H, Yasufuku N, et al. Three-dimensional limit analysis of seismic displacement of slope reinforced with piles[J]. Soil Dynamics and Earthquake Engineering, 2015, 77:446~452. doi: 10.1016/j.soildyn.2015.06.015 [27] Zhang Y B, Chen G Q, Zheng L, et al. Effects of near-fault seismic loadings on run-out of large-scale landslide:A case study[J]. Engineering Geology, 2013, 166:216~236. doi: 10.1016/j.enggeo.2013.08.002 [28] Zhang Y B, Wang J M, Xu Q, et al. DDA validation of the mobility of earthquake-induced landslides[J]. Engineering Geology, 2015, 194:38~51. doi: 10.1016/j.enggeo.2014.08.024 [29] Zhang Y B, Zhang J, Chen G Q, et al. Effects of vertical seismic force on initiation of the Daguangbao landslide induced by the 2008 Wenchuan earthquake[J]. Soil Dynamics and Earthquake Engineering, 2015, 73:91~102. doi: 10.1016/j.soildyn.2014.06.036 -





 下载:
下载:






 下载:
下载: