基于应变软化的岩土工程单元安全度评价方法研究

姜谙男; 郑帅; 白涛; 段龙梅; 申发义; 赵亮; 薛焕东

doi:10.12090/j.issn.1006-6616.2018.24.06.086

基于应变软化的岩土工程单元安全度评价方法研究

doi: 10.12090/j.issn.1006-6616.2018.24.06.086

1.
大连海事大学道桥所, 辽宁大连 116026
2.
吉林省交通规划设计院, 吉林长春 130021

基金项目:

国家自然科学基金项目 51678101

中央高校基本科研业务费专项资金 3132014326

详细信息

作者简介:
姜谙男(1971-), 男, 教授, 主要从事隧道稳定性与优化等方面的教学和科研。E-mail:jiangannan@163.com

中图分类号: TU45
计量
- 文章访问数: 153
- HTML全文浏览量: 66
- PDF下载量: 10
- 被引次数: 0
出版历程
- 收稿日期: 2017-07-03
- 修回日期: 2018-08-02
- 刊出日期: 2018-12-01

A STUDY ON EVALUATION METHOD OF GEOTECHNICAL ENGINEERING ZONE SAFETY DEGREE BASED ON STRAIN SOFTENING

1.
Institute of Road and Bridge Engineering, Dalian Maritime University, Dalian 116026, Liaoning, China
2.
Jilin Provincial Communication Planning and Design Institute, Changchun 130021, Jilin, China

摘要

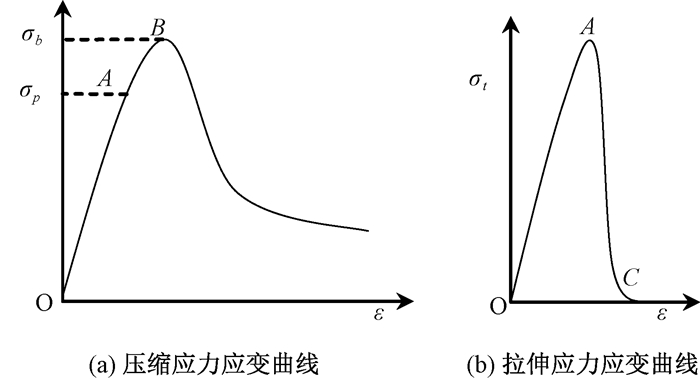
摘要: 针对大多已有岩土工程局部安全评价方法未考虑拉伸破坏和屈服、破坏阶段的问题，对围岩的局部安全评价方法进行了相应的改进。基于Mohr-Coulomb屈服准则和应变软化模型建立了单元安全度的评价方法和新的定义，综合考虑剪切和拉伸破坏模式，定义一个统一的变量ZSD来表征和量化岩土体单元从弹性、屈服到破坏的安全程度，实现复杂应力状态下岩土体渐进破坏过程的局部安全性定量评价。推导了ZSD的各阶段表达公式，利用FISH语言在FLAC^3D平台编写程序。通过相应的实例和工程进行了ZSD计算，验证了该方法的正确性与有效性。该方法具有参数表达简单，易于在程序中实现，可通过ZSD所在值域判断单元所处的状态，可直观揭示岩土体渐进破坏过程等诸多优点。该方法为分析和预测岩土工程中危险区域的演化和描述渐进破坏过程提供了有效的手段。
- 应变软化 /
- 单元安全度 /
- 安全评价 /
- 岩土工程
Abstract: In view of the fact that the problems encountered in the stage of tensile failure, yield and failure stage are not considered in most existing local safety evaluation methods of geotechnical engineering, the local safety evaluation method of surrounding rock has been improved accordingly. The new evaluation method and new definition of geotechnical engineering zone safety degree were established based on strain softening model and Mohr-Coulomb yield criterion. It is defined by a uniform variable ZSD (Zone Safety Degree)synthetically considering shear and tension pattern, describing the safety degree of geotechnical body element from elastic stage, yield stage to failure stage, realizing the local safety quantitative evaluation with complex stress state and progressive failure progress. The ZSD expression formulas at different stages are derived, and the program by FISH language based on FLAC^3D platform is compiled. The corresponding examples and engineering are calculated, and the accuracy and efficiency of the ZSD method are verified. This method is simple in parameter expression and easy to be implemented in the program. It can judge the state of the zone in the range of ZSD and directly reveal the progressive failure process of rock and soil mass. This method provides an effective means for the analysis and prediction of the evolution of hazardous areas in geotechnical engineering and the description of the progressive failure process.
- strain softening /
- zone safety degree /
- safety evaluation /
- geotechnical engineering

HTML全文

图 1 典型的岩石应力应变曲线^[13]

Figure 1. Typical stress-strain curves of rock^[13]

下载: 全尺寸图片幻灯片

图 2 应变软化模型线弹性卸载

Figure 2. Linear elastic unloading of the strain softening model

下载: 全尺寸图片幻灯片

图 3 弹性阶段应力状态分析图

Figure 3. Stress state analysis in the elastic stage

下载: 全尺寸图片幻灯片

图 4 边坡计算结果对比图

Figure 4. Comparison of the calculation results of the slope

下载: 全尺寸图片幻灯片

图 5 滑移面附近的单元安全度等值线

Figure 5. The ZSD contours near the shear sliding surface

下载: 全尺寸图片幻灯片

图 6 数值模型与约束条件

Figure 6. The numerical model and constraint conditions

下载: 全尺寸图片幻灯片

图 7 巴西圆盘的ZSD等值线图

Figure 7. ZSD contours of the Brazilian disk

下载: 全尺寸图片幻灯片

图 8 隧道数值模型与支护结构

Figure 8. Numerical model and lining structure of the tunnel

下载: 全尺寸图片幻灯片

图 9 ZSD等值线图与塑性区图对比

Figure 9. Comparison of ZSD contours and plastic zones

下载: 全尺寸图片幻灯片

表 1 ZSD表达式

Table 1. Expression of ZSD

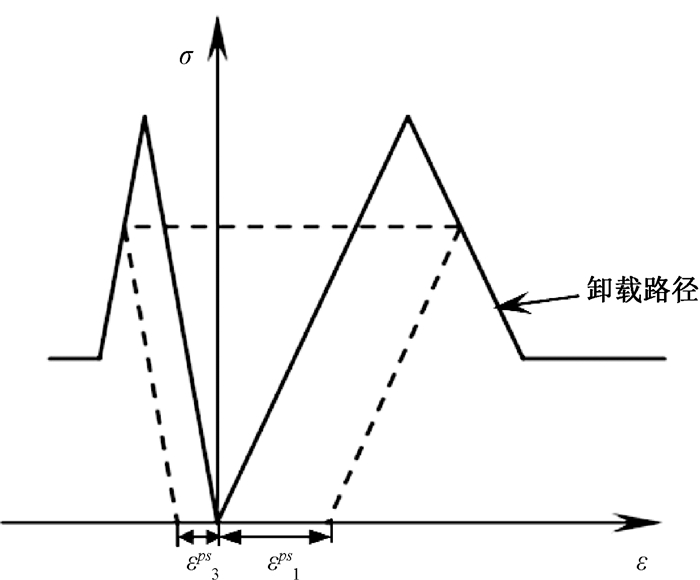
单元状态	弹性阶段		屈服阶段			破坏阶段
判断标准	ε^ps=0, ε^pt=0 (σ₁+σ₃)/2 < σ₀ (剪切)	ε^ps=0, ε^pt=0 (σ₁+σ₃)/2≥σ₀ (拉伸)	0 < ε^ps≤ε^ps ε^pt=0 (剪切)	ε^ps=0 0 < ε^pt≤ε^pt (拉伸)	0 < ε^ps≤ε^ps 0 < ε^pt≤ε^pt (剪切和拉伸)	ε^ps>ε^ps (剪切)	ε^pt>ε^pt (拉伸)
ZSD函数	公式(7)	公式(8)	公式(9)	公式(10)	公式(11)	公式(9)	公式(10)
ZSD性质	ZSD∈[1, +∞)+∞该段最安全状态1进入屈服状态	ZSD∈[1, +∞)+∞该段最安全状态1进入屈服状态	ZSD∈[0, 1)1进入屈服状态0进入破坏状态	ZSD∈[0, 1)1进入屈服状态0进入破坏状态	ZSD∈[0, 1)1进入屈服状态0进入破坏状态	ZSD∈[0, -∞)值越小，破坏程度越高	ZSD∈[0, -∞)值越小，破坏程度越高
单调性	ZSD值随安全性的降低而单调递减

下载: 导出CSV

参考文献(19)

[1]	郑颖人, 邱陈瑜, 张红, 等.关于土体隧洞围岩稳定性分析方法的探索[J].岩石力学与工程学报, 2008, 27(10):1968~1980. doi: 10.3321/j.issn:1000-6915.2008.10.003 ZHENG Yingren, QIU Chenyu, ZHANG Hong, et al. Exploration of stability analysis methods for surrounding rocks of soil tunnel[J]. Chinese Journal of Rock Mechanics and Engineering, 2008, 27(10):1968~1980. (in Chinese with English abstract) doi: 10.3321/j.issn:1000-6915.2008.10.003
[2]	崔玉龙, 邓建辉.某水电工程边坡开挖及支护过程稳定性分析[J].水利水电技术, 2017, 48(12):188~194. http://d.old.wanfangdata.com.cn/Periodical/slsdjs201712030 CUI Yulong, DENG Jianhui. Stability analysis on slope of a hydropower project during excavation and supporting[J]. Water Resources and Hydropower Engineering, 2017, 48(12):188~194. (in Chinese with English abstract) http://d.old.wanfangdata.com.cn/Periodical/slsdjs201712030
[3]	Hoek E, Bray J W. Rock slope engineering[M]. 3rd ed. London:Institute of Mineral and Metallurgy, 1981, 309.
[4]	周辉, 张传庆, 冯夏庭, 等.隧道及地下工程围岩的屈服接近度分析[J].岩石力学与工程学报, 2005, 24(17):3083~3087. doi: 10.3321/j.issn:1000-6915.2005.17.013 ZHOU Hui, ZHANG Chuanqing, FENG Xiating, et al. Analysis of rock mass stability in tunnel and underground engineering based on yield approach index[J]. Chinese Journal of Rock Mechanics and Engineering, 2005, 24(17):3083~3087. (in Chinese with English abstract) doi: 10.3321/j.issn:1000-6915.2005.17.013
[5]	蓝航.基于FLAC^3D的边坡单元安全度分析及应用[J].中国矿业大学学报, 2008, 37(4):570~574. doi: 10.3321/j.issn:1000-1964.2008.04.026 LAN Hang. Analysis of zone safety degree of slopes and its application based on FLAC^3D[J]. Journal of China University of Mining and Technology, 2008, 37(4):570~574. (in Chinese with English abstract) doi: 10.3321/j.issn:1000-1964.2008.04.026
[6]	李树忱, 李术才, 徐帮树.隧道围岩稳定分析的最小安全系数法[J].岩土力学, 2007, 28(3):549~554. doi: 10.3969/j.issn.1000-7598.2007.03.022 LI Shuchen, LI Shucai, XU Bangshu. Minimum safety factor method for stability analysis of surrounding rockmass of tunnel[J]. Rock and Soil Mechanics, 2007, 28(3):549~554. (in Chinese with English abstract) doi: 10.3969/j.issn.1000-7598.2007.03.022
[7]	万世明, 吴启红, 谢飞鸿, 等.基于JRC-JCS模型的边坡局部稳定性分析[J].中南大学学报(自然科学版), 2014, 45(4):1227~1231. http://2010.cqvip.com/QK/90745B/201404/49725560.html WAN Shiming, WU Qihong, XIE Feihong, et al. Local stability analysis for slope based on JRC-JCS model[J]. Journal of Central South University (Science and Technology), 2014, 45(4):1227~1231. (in Chinese with English abstract) http://2010.cqvip.com/QK/90745B/201404/49725560.html
[8]	蒋青青, 胡毅夫, 赖伟明.层状岩质边坡遍布节理模型的三维稳定性分析[J].岩土力学, 2009, 30(3):712~716. doi: 10.3969/j.issn.1000-7598.2009.03.025 JIANG Qingqing, HU Yifu, LAI Weiming, Three-dimensional stability analysis of stratified rock slope based on ubiquitous-joint model[J]. Rock and Soil Mechanics, 2009, 30(3):712~716. (in Chinese with English abstract) doi: 10.3969/j.issn.1000-7598.2009.03.025
[9]	丁丽宏, 马强.基于组合权重的模糊物元模型在边坡稳定性评价中的应用[J].地震工程学报, 2017, 39(5):946~950. doi: 10.3969/j.issn.1000-0844.2017.05.0946 DING Lihong, MA Qiang. Application of fuzzy matter-element model Based on coefficients of combined weights to evaluate slope stability[J]. China Earthguake Engineering Journal, 2017, 39(5):946~950. (in Chinese with English abstract) doi: 10.3969/j.issn.1000-0844.2017.05.0946
[10]	王晓健, 李同春, 何金文, 等. IBE-PFE方法在某水电站泄洪洞出口边坡稳定分析中的应用[J].水利水电技术, 2017, 48(3):140~145. http://d.old.wanfangdata.com.cn/Periodical/slsdjs201703025 WANG Xiaojian, LI Tongchun, HE Jinwen, et al. Application of IBE-PFE method to analysis on stability of slope around flood discharge tunnel outlet of a hydropower station[J]. Water Resources and Hydropower Engineering, 2017, 48(3):140~145. (in Chinese with English abstract) http://d.old.wanfangdata.com.cn/Periodical/slsdjs201703025
[11]	王晓鸿, 王家来, 梁发云.应变软化岩土材料内扩孔问题解析解[J].工程力学, 1999, 16(5):71~76. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=QK199900122965 WANG Xiaohong, WANG Jialai, LIANG Fayun. Analytical solution to expansion of cavity in strain-softening materials[J]. Engineering Mechanics, 1999, 16(5):71~76. (in Chinese with English abstract) http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=QK199900122965
[12]	金丰年, 钱七虎.岩石的单轴拉伸及其本构模型[J].岩土工程学报, 1998, 20(6):5~8. doi: 10.3321/j.issn:1000-4548.1998.06.003 JIN Fengnian, QIAN Qihu. Uniaxial tension and mechanical model of rock[J]. Chinese Journal of Geotechnical Engineering, 1998, 20(6):5~8. (in Chinese) doi: 10.3321/j.issn:1000-4548.1998.06.003
[13]	马春景.考虑流-固耦合与时间效应影响的隧道稳定性分析[D].大连: 大连海事大学, 2016. http://cdmd.cnki.com.cn/Article/CDMD-10151-1016057073.htm MA Chunjing. The stability analysis of tunnel considering fluid-solid coupling and time effect[D]. Dalian: Dalian Maritime University, 2016. (in Chinese with English abstract) http://cdmd.cnki.com.cn/Article/CDMD-10151-1016057073.htm
[14]	赵星光, 蔡明, 蔡美峰.岩石剪胀角模型与验证[J].岩石力学与工程学报, 2010, 29(5):970~981. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=yslxygcxb201005013 ZHAO Xingguang, CAI Ming, CAI Meifeng. A rock dilation angle model and its verification[J]. Chinese Journal of Rock Mechanics and Engineering, 2010, 29(5):970~981(in Chinese with English abstract)). http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=yslxygcxb201005013
[15]	陆银龙, 王连国, 杨峰, 等.软弱岩石峰后应变软化力学特性研究[J].岩石力学与工程学报, 2010, 29(3):640~648. http://d.old.wanfangdata.com.cn/Periodical/yslxygcxb201003026 LU Yinlong, WANG Lianguo, YANG Feng, et al. Post-peak strain softening mechanical properties of weak rock[J]. Chinese Journal of Rock Mechanics and Engineering, 2010, 29(3):640~648. (in Chinese with English abstract) http://d.old.wanfangdata.com.cn/Periodical/yslxygcxb201003026
[16]	彭文斌. FLAC^3D实用教程[M].北京:机械工业出版社, 2007. PENG Wenbin, Practical tutorial of FLAC3D[M], Beijing:China Machine Press, 2007.
[17]	郑颖人, 叶海林黄润秋.地震边坡破坏机制及其破裂面的分析探讨[J].岩石力学与工程学报, 2009, 28(8):1714~1723. doi: 10.3321/j.issn:1000-6915.2009.08.024 ZHENG Yingren, YE Hailin, HUANG Runqiu. Analysis and discussion of failure mechanism and fracture surface of slope under earthquake[J]. Chinese Journal of Rock Mechanics and Engineering, 2009, 28(8):1714~1723. (in Chinese with English abstract) doi: 10.3321/j.issn:1000-6915.2009.08.024
[18]	郭明伟, 邓琴, 李春光, 等.巴西圆盘试验问题与三维抗拉强度准则[J].岩土力学, 2008, 29(S1):545~549, 554. http://d.old.wanfangdata.com.cn/Periodical/ytlx2008z1110 GUO Mingwei, DENG Qin, LI Chunguang, et al. Brazilian disc test and 3-D tensile strength principle[J]. Rock and Soil Mechanics, 2008, 29(S1):545~549, 554. (in Chinese with English abstract) http://d.old.wanfangdata.com.cn/Periodical/ytlx2008z1110
[19]	Fairhurst C. On the validity of the 'Brazilian' test for brittle materials[J]. International Journal of Rock Mechanics and Mining Sciences & Geomechanics Abstracts, 1964, 1(4):535~546. http://www.sciencedirect.com/science/article/pii/0148906264900609