RHEOLOGIC IMPLICATIONS OF CONJUGATE SHEAR ANGLES
-
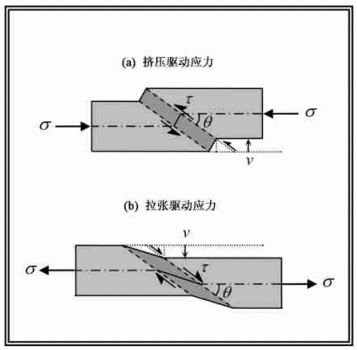
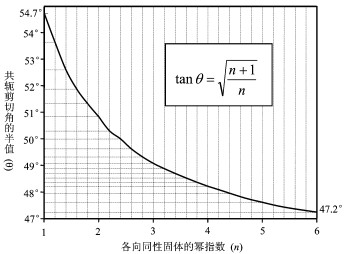
摘要: 基于最大侧向位移速率假设(Maximum lateral displacement rate, 简称MLDR), 本文提出了一个关于共轭剪切角的流变学理论。根据这个假设, 无论是压应力或张应力作用在一个固体上, 剪切带总是沿着使得被剪切带分割的块体的侧向位移速率为最大的方向发育。换句话说, 如果平行于驱动应力的纵向位移速率或驱动应力的大小被看作为边界条件, 那些被剪开的块体总是以最快的可能速度从两侧挤出或饲入变形区。该理论的优点是:剪切位移的方向是可逆的。因此, 同一剪切带可以在挤压和拉张应力体系中活动。在各向同性固体中, 剪切带的方位和驱动应力方向之间的夹角θ由方程式 tan θ=
-
关键词:
- 共轭剪切角 /
- 最大侧向位移速率假设 /
- 流变学
Abstract: A rheologic theory on conjugate shear angles is proposed based on the maximum lateral displacement rate (MLDR)hypothesis, which states that when compressive or tensile stress is applied to a solid, shear bands are formed in the solid in the orientations that give rise to the maximum lateral displacement rates of the blocks divided by the shear bands.In other words, it is postulated that the sheared blocks are laterally extruded from or fed into the deformation domain at the greatest possible velocity.The merit of this theory is:the sense of shear displacement is reversible.Hence, the same shear bands can be activated in both compressive and tensile stress regimes.In an isotropic model, the angle θ between the driving stress and the shear bands formed is determined by the equation, tan θ=-
Key words:
- conjugate shear angle /
- maximum lateral displacement rate /
- rheology
-
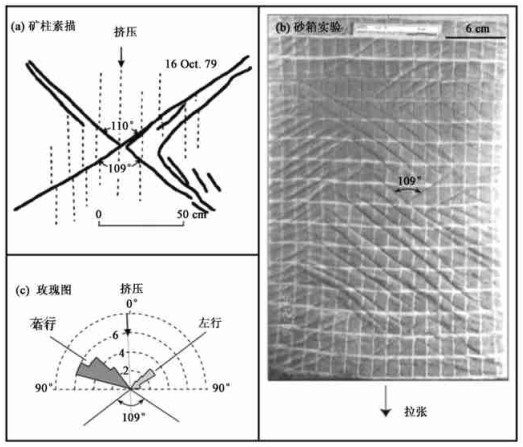
图 1 (a) 粗实线描绘地下1000m深处的矿柱上观察到的共轭剪切带, 其共轭角为109°和110°(Boulby煤矿, 克莱福兰, 英国)。垂直虚线指示挖矿用的铲子留下的沟痕, 它们已被沿着剪切带错开(据Watterson, 1999)[1]。(b)用于模拟伸展构造的砂箱实验中观察到的共轭剪切带, 砂层厚为3cm, 用常拉伸速率5×10-3 cm/sec, 通过一可伸长的弹性底层拉至46%的总伸展量。模拟实验的尺度比设计为约10-5, 模型中的1cm代表自然界中的1km。当伸展量超过20%时, 砂箱顶面出现图中所示的共轭剪切带, 测得其共轭角为109°(据Bahroudi et al., 2003)[3]。(c)加拿大西大省晚太古代花岗绿岩中发育的共轭剪切带方位的玫瑰图, 其共轭角也为109°(据Park, 1981)[4]。
Figure 1. (a)Shear bands (solid lines)with conjugate angles of 109°and 110°in a roof support pillar at depth of 1000 m, Boulby mine, Cleveland, UK.Vertical broken lines indicate grooves made by excavator shovel, which are offset along yield bands (after Watterson, 1999)[1].(b)Conjugate shear bands obtained in sandbox analogue modeling of extensional structures using a sand layer 3 cm thick extended at constant rate about 5 ×10-3 cm sec by a stretchable basement to a total extension of 46%(the length ratio of analogue modeling is designed to be about 10-5, implying that 1 cm in the model simulates 1km in nature).The conjugate shear bands with conjugate angles of 109°in the top view of sandbox merge as the amount of extension exceeds 20%(after Bahroudi et al., 2003)[3].(c)Rose diagram showing the orientations of conjugate shear bands measured at 15°intervals, in the later Archaean granite-greenstone terrain of the Western Superior Province, Canada, with a conjugate angle of 109°(after Park, 1981)[4].
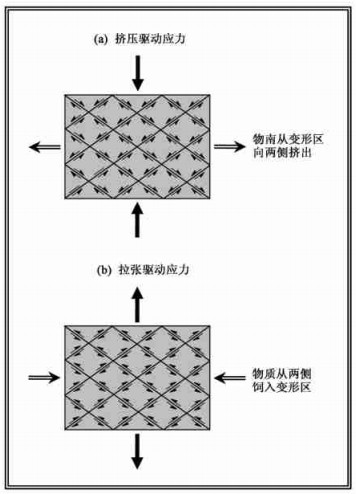
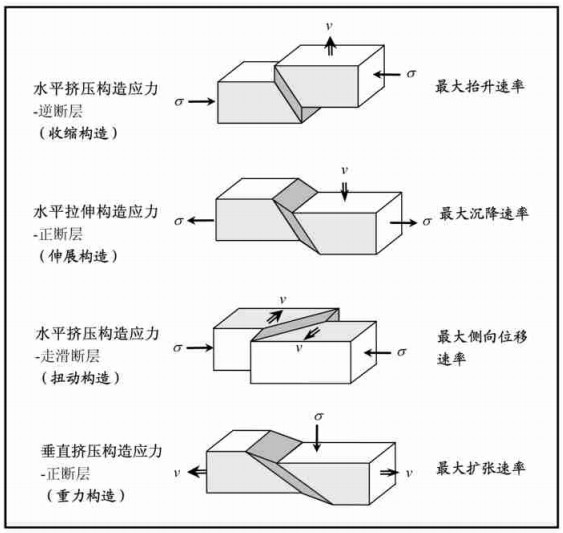
图 2 描述最大侧向位移速率假设(MLDR)的示意图(a)挤压驱动应力情形; (b)拉张驱动应力情形。M LDR假设意味着让被剪切带切割的块体以最快的速度从变形区逃逸出来(在挤压情形, 称为挤出作用)或以最快的速度饲入变形区(在拉张情形, 称为颈缩作用)。
Figure 2. Diagrams showing the hypothesis of maximum lateral displacement rate (MLDR) in the cases of (a) compressive and (b)tensile driving stress applied to a solid.The MLDR hypothesis implies the fastest way for the blocks divided by conjugate shear bands to be laterally extruded from the deformation domain in the case of compressive driving stress, known as extrusion, or to be laterally fed into the deformation domain in the case of tensile driving stress, known as necking.
图 3 一个剪切带模型, 用于计算一个均质、各向同性固体受(a)挤压驱动应力和(b)拉张驱动应力作用时的侧向位移速率。
Figure 3. A shear band model to calculate the lateral displacement rate of a homogeneous and isotropic solid deformed by (a)compressive driving stress and (b)tensile driving stress (σ).
图 4 各向同性固体中剪切带的共轭钝角的半值(θ)随其幂指数(n)的变化关系
Figure 4. Variations of the half conjugate angle of shear bands, θ, with the power-law index, n, in an isotropic solid
-
[1] Watterson J.The future of failure:stress or strain?[J].Journal of Structural Geology, 1999, 21:939~948. doi: 10.1016/S0191-8141(99)00012-7 [2] Ramsay JG.Shear zone geometry:a review[J]. Journal of Structural Geology, 1980, 2:83~89. doi: 10.1016/0191-8141(80)90038-3 [3] Bahroudi A, Koyi HA, Talbot CJ.Effect of ductile and frictional decollements on style of extension[J].Journal of Structural Geology, 2003, 25:1401~1423. doi: 10.1016/S0191-8141(02)00201-8 [4] Park RG.Shear-zone deformation and bulk strain in granite-greenstone terrain of the Western Superior Provinces, Canada[J]. Precambrian Research, 1981, 14:31~47. doi: 10.1016/0301-9268(81)90034-6 [5] Robertson EC. Viscoelasticity of Rocks. In: State of Stress in the Earth' s Crust, W. R. Judd (ed. )[M]. New York: Elsevier, 1964. 181~233. [6] Zhang Y. Rheologic implications of the geometry of ductile shear zones. In: Maximum Lateral Displacement Rate Theory, Chapter 2. Nanjing, 2005, 123p. [7] Zhang Y. Rheologic implications of the geometry of low-angle normal faults. In: Maximum Lateral Displacement Rate Theory, Chapter 3. Nanjing, 2006, 123p. -
下载:
计量
- 文章访问数: 250
- HTML全文浏览量: 103
- PDF下载量: 10
- 被引次数: 0
