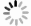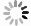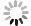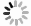THEORETICAL DEVELOPMENT OF MAXIMUM EFFECTIVE MOMENT CRITERION
-
摘要: 在分析"导致变形带内先存面理或层理发生转动的最大有效力矩与先存面理或层理方向有关"的基础上, 对最大有效力矩准则(Meff=0.5(σ1-σ3) Lsin2αsinα)进行理论上的拓展, 提出了可以判定任意方向先存面理最大有效力矩的准则——泛最大有效力矩准则(MG-eff=0.5(σ1-σ3)Lsin2αsin(α-θ)), 其中当先存面理与最大主压应力(σ1)平行时, 则成为最大有效力矩准则。该准则的理论分析表明:①当先存面理与σ1平行时, 在σ1左右两侧±54.7°方向出现2个有效力矩的最大值, 形成共轭的变形带, 钝角(109.4°)对着σ1方向; ②当先存面理与σ1斜交时, 在σ1的另一侧出现1个有效力矩的最大值, 从而只出现一个方向的变形带, 并随着先存面理偏离σ1方向, 变形带与σ1的夹角逐渐减小(从θ=0°时的54.7°, 减小到θ=90°时的35.3°), 而与先存面理之间的夹角逐渐增大(从θ=0°时的54.7°, 增加到θ=90°时的125.3°); ③当先存面理与σ1垂直时, 在σ1左右两侧± 35.3°方向出现2个有效力矩的最大值, 也形成共轭的变形带, 但锐角(70.6°)对着σ1方向。在主应变平面上变形带与先存面理方向及变形带剪切方向(左旋或右旋)已知的情况下, 可以确定最大主压应力方向。泛最大有效力矩准则克服了最大有效力矩准则与滑移线理论不相容的问题, 可以解释大多膝褶带非共轭发育等多种现象, 预期在韧性变形域中具有广阔的应用前景。Abstract: Theoretical analysis shows that the Maximum Effective Moment, which cause preexisting cleavage or bedding to rotate, is related to the direction of pre-existing cleavage or bedding, and the Maximum Effective Moment Criterion (Meff=0.5(σ1-σ3) Lsin2αsinα, simplified as MEMC) proposed by Zheng et al is theoretically expanded to General Criterion of Maximum Effective Moment (MG-eff=0.5(σ1-σ3) Lsin2αsin (α-θ), simplified as GCMEM), which can be used to determine the Maximum Effective Moment with any direction of cleavage in this paper.MEMC is a special case of GCMEM when cleavage is parallel to maximum principal compressive stress (σ1).Theoretical analysis of GCMEM shows that:① when cleavage is parallel to σ1, there occur two values of Maximum Effective Moment symmetrically on either side of σ1 in the direction of ± 54.7°, and two conjugate deformation zone are predicted to appear with obtuse angle (109.4°) facing σ1 direction.② When cleavage is oblique to σ1, one Maximum Effective Moment, along which one deformation zone will appear, is predicted to occur on other side of σ1, and the angle between deformation zone and σ1 will decrease (from 54.7 ° when θ=0° reduced to 35.3° when θ=90°), while the angle between pre-existing cleavage and deformation zone will increase (from 54.7° when θ=0° increased to 125.3° when θ=90°) with pre-existing cleavage deviating from the σ1 direction.③ when cleavage is perpendicular to σ1, there also occur two values of Maximum Effective Moment symmetrically on either side of σ1 in the direction of ± 35.3°, but two conjugate deformation zone with acute angle (70.6°) facing σ1 direction.When the directions of pre-existing cleavage and deformation zone on principal strain surface and shear direction (sinistral or dextral) are known, the direction of maximum principal stress can be determined.GCMEM overcomes the incompatibility of MEMC with Slip Line Theory, and can be used to explain most of the kink zone development and other non-conjugate phenomena.It is expected to have wide application prospects in ductile deformation field.
-
Key words:
- Maximum Effective Moment /
- ductile deformation /
- pre-existing cleavage /
- deformation zone /
- conjugate
-
0. 引言
变形带内"先存面理或层理"(以下均用"先存面理"表述)发生转动是变形带的基本特征, 该转动与力矩有关[1]。郑亚东等[1~2]根据这一思想, 在定量分析共轭膝褶带[3]和共轭伸展褶劈理交角[4]的基础上, 提出了岩石变形的最大有效力矩准则, 即Meff=0.5(σ1-σ3) Lsin2αsinα, 其中(σ1-σ3)为材料屈服时的差应力, L为σ1(最大主压应力)方向的单位长度, α为韧性变形带与最大主压应力(σ1)间的夹角, 表明最大有效力矩出现在主应力轴(σ1)左右两侧54.7°方向, 54.7° ± 10°无显著力矩降, 构成了韧性变形带形成的有利区间。该准则在实践中可解释膝褶带、伸展褶劈理、高盐层中的屈服带、低角正断层、高角逆断层、结晶基底中的菱网状剪切带等的形成[1~2], 解决了低角正断层[5~8]和高角度逆断层的产状与摩尔-库伦准则不相容的问题[9~11]。有效力矩概念的提出为韧性变形力学机理的研究提供了新的途径[12], 是地质学基础理论的一大贡献。
然而, 更深入的研究分析表明, 郑亚东等提出的有效力矩准则[1]存在一些问题。根据郑亚东等[1]的定义, α是σ1和面理的夹角, 而θ是σ1和面理法线的夹角(见图 1)[1]。按照这样的定义, 必然会出现以下3个结果:①潜在变形带方向为τα方向, 与面理方向(根据角度α和θ定义也是τα方向)是平行的, 而潜在变形带若和面理平行, 面理的有效力臂就为零, 有效力矩也就为零, 这显然是不合理的; ②根据角度的定义, α和θ不是独立的, 它们互为余角(见图 1); ③一旦面理方向确定后, α(包括θ)是确定值, 并不是变量, 按有效力矩公式(Meff=0.5(σ1-σ3) Lsin2αsinα)计算出的有效力矩也是一个确定值, 而不是变量, 因此, 不存在所谓的最大值(最大有效力矩值)。通过对文献[1]的整体分析, 上述角度定义中隐含的这3个结果, 既非文献[1]的本意, 也不合理, 显然存在问题。
图 1 最大有效力矩准则力学分析示意图[1]α-σ1与面理的夹角; θ-σ1与面理法线的夹角; H-有效力臂长度; L-σ1方向的单位长度Figure 1. A sketch map of mechanical analysis of the Maximum Effective Moment Criterion从力学上很容易理解, 力矩是针对转动而言的, 而转动是有对象的。文献[4]里的转动对象是指先存面理。根据文献[1]中受力分析图(见图 1)标明的"有效力臂"为"H"、有效力矩Meff的计算公式以及有效力矩随α的变化这3方面的情况, 可以推定, 先存面理方向为最大主压应力方向。因为, 只有在先存面理方向和最大主压应力方向平行的条件下, 才能满足:①先存面理"有效力臂"H=Lsinα; ②潜在变形带与最大主压应力的夹角为α, 沿潜在变形带的剪应力为0.5(σ1-σ3) sin2α; ③有效力矩Meff (力×有效力臂)为0.5(σ1-σ3) Lsin2αsinα; ④ α是可以变化的, 有效力矩存在最大值。
通过上述分析, 文献[1]的有效力矩计算中隐含了"先存面理方向和最大主压应力方向平行"的条件。只有满足这一条件, 最大有效力矩的计算公式才是正确的。然而这种隐含显然是不太合理的。因为虽然自然界中先存面理与最大主压应力平行的情况很多(如水313地质力学学报2011平地层遭受挤压), 但先存面理毕竟可以是各种方向的, 最大主压应力也可以是各种方向的, 理论上它们之间可以以任何角度相交。这是文献[1]存在问题的根源所在。
很容易理解, 先存面理方向的改变, 会导致有效力臂大小的改变, 并导致最大有效力矩值的变化, 即最大有效力矩(导致先存面理沿变形带方向发生转动)与先存面理的方向有关。如:与σ1夹角为45°的先存面理发生转动的最大有效力矩出现在主应力轴(σ1)另一侧45°方向(该方向力(剪应力)和有效力臂都达到最大值)。
另外, 根据塑性力学的滑移线理论[13], 平面应变情况下共轭滑移线夹角的交角为45°[14]; 单侧拉伸情况下, 沿拉伸方向(σ3)的共轭夹角为109.4°, 即缩短方向为70.6°[13]; 野外观测表明, 很多膝褶带并非共轭发育[15~16]。这些情况均与现有的最大有效力矩准则不一致。
本文在理论研究分析的基础上, 应用郑亚东等力矩分析的思想[1], 对其提出的最大有效力矩准则进行了理论拓展, 提出了可以判定任意方向先存面理或层理发生转动的最大有效力矩准则---泛最大有效力矩准则。泛最大有效力矩准则表明:①郑亚东等提出的最大有效力矩准则是泛最大有效力矩准则在先存面理或层理与σ1平行情况下的一个特例; ②不同方向先存面理达到最大有效力矩的方位及组合是不一致的, 如与σ1间的夹角为0°、45°、90°的先存面理发生转动的最大有效力矩分别出现在σ1两侧54.7°(两组, 共轭)、另一侧45°方向(一组, 非共轭)和两侧35.3°(两组, 共轭); ③克服了最大有效力矩准则与滑移线理论不相容的问题, 可以解释大多膝褶带非共轭发育等多种现象。该成果预期在韧性变形域中具有广阔的应用前景。
1. 不同方向先存面理有效力矩的计算
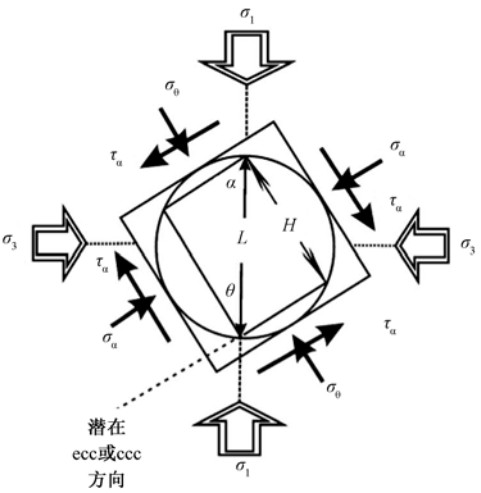
如图 2所示, 设先存面理与σ1间的夹角为θ(AC与σ1的夹角, 逆时针为正, AC为先存面理方向), BC为可能的变形带方向, 与σ1间夹角为α, AC为先存面理的单位长度(AC=L), 则AC沿BC面发生转动的有效力矩(Meff)为:
图 2 不同方位先存面理有效力矩计算参数示意图AC的延伸方向为先存面理方向, 先存面理与σ1的夹角为θ(从σ1到先存面理, 逆时针为正), 变形带(BC方向)与σ1的夹角为α(从σ1到先存面理, 逆时针为正), AC为先存面理的单位长度L。单位长度先存面理沿变形带剪切弯曲的力臂为AB (H), 有效力矩为AB (H=Lsin (α-θ))与沿变形带剪应力(0.5(σ1-σ3) sin2α)的乘积(0.5(σ1-σ3) Lsin2αsin (α-θ))Figure 2. Calculating parameters of effective moment of pre-exiting cleavages with different directions
(1) (1) 式中, (σ1-σ3)为材料屈服时的差应力, L为先存面理的单位长度。它们的涵义与最大有效力矩准则[1~2]中的一致。
容易看出, 当θ为0时, (1)式就成为郑亚东等[1~2]提出的最大有效力矩准则的表达式, 即已有的最大有效力矩准则[1~2]是拓展后的有效力矩准则在先存面理与σ1平行状态下的特例。
根据上述情况, 再考虑到郑亚东等[1~2]提出的最大有效力矩准则已被大家所熟知, 本文把拓展后的最大有效力矩准则称为"泛最大有效力矩准则"(General Criterion of Maximum Effective Moment, 简称GCMEM)。
2. 不同方向先存面理出现最大有效力矩的方位及变化规律
对于确定性质的材料而言, 材料屈服时的差应力和先存面理的单位长度都是确定的[1]。这样, 只要确定任意先存面理方向(由θ值确定, 见图 2)有效力矩分量sin2αsin(α-θ)的最大值(取绝对值), 就可以确定该先存面理有效力矩的最大值(取绝对值)。为此只要讨论有效力矩分量sin2αsin (α-θ)值的变化情况, 就可以确定有效力矩的变化情况。在没有特别说明的情况下, 以下均由有效力矩的分量sin2αsin (α-θ)来代表有效力矩值。
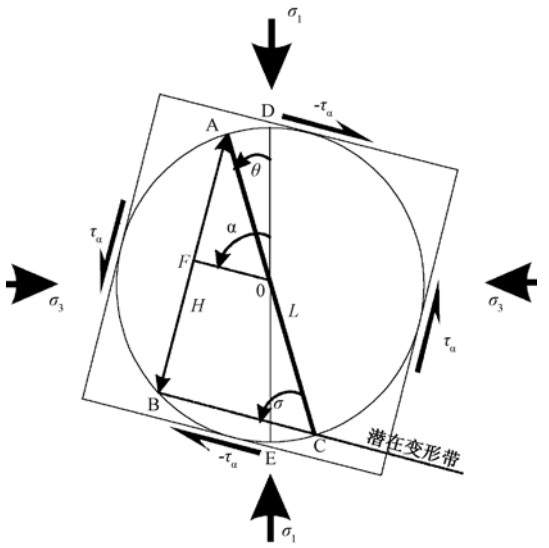
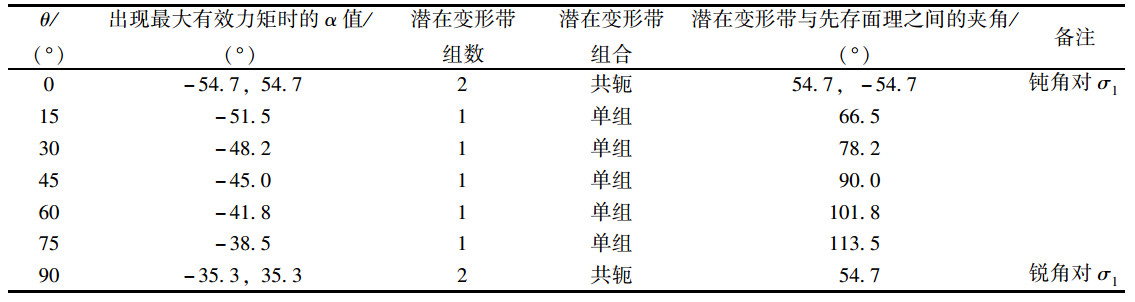
由于θ在90°~180°和0~90°的变化是对称的, 为此, 只要编制θ在0~90°变化时sin2αsin (α-θ)的等值线图(见图 3, α在0~180°变化, 图中数值的符号代表力矩的方向, 顺时针转动为正, 逆时针转动为负, 下同), 可以反映任意先存面理出现最大有效力矩的方位及其变化。同时编制θ分别为0°, 15°, 30°, 45°, 60°, 75°, 90°时, sin2αsin(α-θ)的分布图(见图 4, α在0~180°变化), 来说明上述特征方位的先存面理出现最大有效力矩的方位。
图 3 不同方位先存面理沿不同方向的有效力矩分量sin2αsin (α-θ)的等值线图a, b, c为不同方位先存面理sin2αsin (α-θ)值的极值分布线, 每一方位的先存面理都有3个极值点, 其中a中的A点(θ=90°)、b中的B点(θ=0°)以及c上的所有点(sin2αsin (α-θ))的绝对值都是最大值, 这样, 当θ=90°或θ=0°时, 有2个最大值, 其他的都只有1个最大值。图中数值的符号代表力矩的方向, 顺时针转动为正, 逆时针转动为负Figure 3. Contour map of the effective moment component of pre-exiting cleavages with different directions
图 4 7个特征方位的先存面理的有效力矩分量sin2αsin(α-θ)与α变化关系及最大值分布图Figure 4. Relationship between sin2αsin(α-θ) and α and the distributions of their maximum values从图 3和图 4可以看出, 每个方位的先存面理在α于0~180°变化时, 有效力矩都有3个极值点。但除了θ为0°或90°(先存面理与σ1平行或垂直)这两种情况下3个极值点中的2个极值点的绝对值相等(均为0.77, 2个都成为最大值)外, 所有其他情况(0° < θ < 90°)的3个有效力矩极值点中, 只有一个绝对值最大, 达到最大值, 即当0° < θ < 90°时, 有效力矩的最大值只有1个。如:θ为45°时, 对应的3个有效力矩的极值(及相应的α值)分别为-0.275(22.5°), 0.275(67.5°), -1.0(135°), 其中只有1个(第3个)是最大值。
当θ为0°(先存面理与σ1平行)时, 有效力矩的最大值有2个(为0.77), 分别出现在σ1轴两侧, 对称分布, α=± 54.7°(见图 3和图 4, α=-54.7°与α=125.3°是同一方位)。预测出现2个共轭的变形带, 与σ1轴的夹角为α=54.7°, 钝角(109.4°)对σ1方向。
随着先存面理偏离σ1方向(θ值不断增加, 0° < θ < 90°), 其中的一个极值点其绝对值不断增加, 到α=45°时达到最大(绝对值从0.77增加到1.0), 之后不断减小(绝对值从1.0减小到0.77);另一个极值点的绝对值不断减小(绝对值从0.77减小到0);第3个极值点的绝对值不断增加(绝对值从0增加到0.77)。3个极值中最大值(绝对值)只有1个(见图 3、图 4)。同时, 随着先存面理偏离σ1方向(θ值不断增加, θ < 90°), 出现最大有效力矩的方位与σ1轴的夹角不断减小(从54.7°降低到35.3°)。当θ=45°时, 最大值达到最大(α=-45°时, 有效力矩达到所有方位最大值中的最大值), 绝对值为1.0。表明, 当0° < θ < 90°时, 只能出现一个方向的变形带(沿有效力矩最大值的方向), 而不是出现共轭变形带。
当θ为90°(先存面理与σ1垂直)时, 有效力矩的最大值也有2个, 分别出现在σ1轴两侧, 对称分布, 但α=± 35.3°。预测也出现2个共轭的变形带, 但与σ1轴的夹角为α=35.3°, 锐角(70.6°)对主压应力方向(σ1)。
在应力状态和先存面理力学性质一致的条件下, 当先存面理与σ1呈45°夹角(θ=45°)时, 最大有效力矩达到最大值(1.0), 沿潜在变形带(先存面理的垂直方向, α=-45°)发生变形相对最为容易; 当先存面理与σ1平行或垂直时(θ=0°或θ=90°), 最大有效力矩达到最小值(0.77), 沿潜在变形带(两组共轭的变形带)发生变形相对最不容易。
上述分析表明, 不同方位先存面理出现最大有效力矩的方位及组合方式是不一致的(见表 1)。当先存面理与σ1平行时, 出现2组共轭的变形带, 它们与σ1的夹角为54.7°, 钝角对主压应力方向, 变形带与先存面理之间的夹角为± 54.7°; 当先存面理与σ1垂直时, 也出现2组共轭的变形带, 它们与σ1的夹角为35.3°, 锐角对σ1方向, 变形带与先存面理之间的夹角为125.3°和54.7°; 当先存面理与σ1既不平行也不垂直时, 只出现1组变形带, 并随着先存面理偏离σ1方向, 变形带与σ1的夹角逐渐减小(从θ=0°时的54.7°, 减小到θ=90°时的35.3°), 而与先存面理之间的夹角逐渐增大(从θ=0°时的54.7°, 增加到θ=90°时的125.3°)(见表 1)。
表 1 不同方位先存面理出现最大有效力矩的方位及其组合Table 1. Directions and potential deformation zones of the maximum effective moment of the different cleavages
3. 变形带方向与最大主压应力(σ1)方位的确定
3.1 变形带方向的确定
泛有效力矩准则的理论分析表明, 出现最大有效力矩的方位(即潜在变形带的方位)不仅与σ1的方位有关, 而且与先存面理的方位有关。变形带方向与σ1方向的夹角是随先存面理的方位而变化的(见表 1), 可以通过泛最大有效力矩准则定量确定。这样, 在已知σ1和先存面理方向的情况下, 可以确定变形带的方向及其组合(见图 5)。值得注意的是:①变形带与主压应力之间的夹角是变化的; ②在大部分情况(先存面理与σ1斜交, 即非平行或垂直)下, 只出现一个方向的变形带, 只有在2种特殊情况(先存面理与σ1方向平行或垂直)下, 才会出现2组共轭的变形带(见图 5)。
图 5 5个特征方位先存面理所对应的变形带及剪切方向关系图(虚线为先存面理方向)Figure 5. Relationship between deformation zone and shear direction of different pre-exiting cleavages3.2 最大主压应力(σ1)方位的确定
由于变形带方向与σ1方向的夹角是随先存面理的方位而变化的, 故不能简单地利用变形带的方位来确定σ1的方位。
泛有效力矩准则的理论分析表明, 利用变形带确定σ1方向的方法如下:在确定变形带剪切方向(左旋或右旋)的基础上, 再在主应变平面(垂直面理、并与滑动剪切方向平行的面)上测量变形带与先存面理之间的夹角, 其中测量方式要根据变形带的剪切方向来确定。当变形带为左旋剪切时, 从变形带起逆时针方向到先存面理, 测量它们之间的夹角ϕ; 当变形带为右旋剪切, 从变形带起顺时针方向测量ϕ(见图 6)。最后利用泛最大有效力矩准则制作的模板确定σ1方向与变形带之间的夹角α(见表 1), 从而确定σ1方向(见图 6)。其中σ1与变形带剪切方向必须是协调的(σ1(矢量)与同侧的剪切滑动方向(矢量)的夹角为锐角, 并且σ1位于先存面理和变形带之间, 见图 5、图 6)
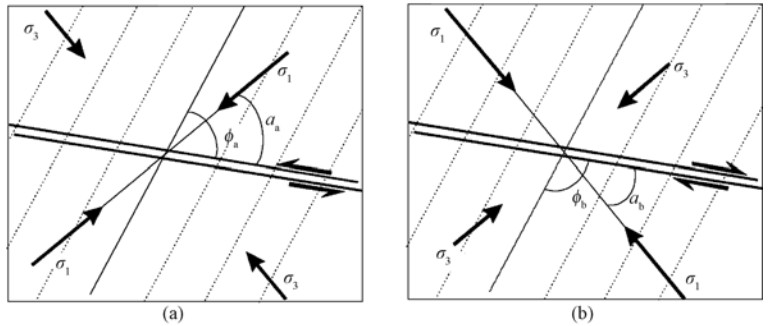
图 6 利用先存面理与变形带间夹角ϕ及变形带剪切方向确定主压应力方位示意图a-变形带左旋剪切, 从变形带起逆时针方向测量ϕ(为ϕa), 并计算确定α(αa), 从而确定σ1方向; b-变形带右旋剪切, 从变形带起顺时针方向测量ϕ(为ϕb), 并计算确定α(αb), 从而确定σ1方向; a和b中先存面理与变形带的方向一致, 但变形带的剪切方向不一致, 结果导致σ1方向相差90°(ϕb-ϕa=90°); 虚线为先存面理方向Figure 6. Principle compressive stress direction determined by the angle ϕ and the shear direction of deformation zone泛有效力矩的理论分析表明, 当先存面理和变形带方向一致, 而变形带剪切方向相反时, 结果会导致σ1方向相差90°(见图 6)。如:图 5a旋转90°(σ1由竖直方向变为水平方向)后, 先存面理和变形带的方向与图 5e都完全一致, 但剪切方向相反, 结果, 它们之间的σ1方向相差90°。
4. 讨论
理论研究分析表明, 郑亚东等[1]提出的最大有效力矩准则是有条件的, 只在先存面理与最大主压应力方向平行时成立。本文通过理论分析和推导, 把该最大有效力矩准则从理论上进行了拓展, 提出了适合任意先存面理方向的新的最大有效力矩准则---泛最大有效力矩准则。最大有效力矩准则是泛最大有效力矩准则在先存面理与最大主压应力平行时的一个特例。泛最大有效力矩准则克服了最大有效力矩准则与塑性力学的滑移线理论[13]不相容的问题, 同时也可以解释自然界出现的大多膝褶带和韧性剪切带非共轭发育[15~17]等多种现象。当然, 泛最大有效力矩准则还有待进一步得到实验的不断验证。
虽然郑亚东等[1]提出的最大有效力矩准则只适用于先存面理或层理平行于最大主压应力方向的变形条件, 但由于先存面理或层理与最大主压应力平行的情况(如水平地层或面理水平的岩层遭受水平挤压)是经常出现的, 该准则还是具有很大的实际应用价值。更重要的是, 郑亚东等[1~2]提出的最大有效力矩准则表明, 用有效力矩分析如膝折等韧性弯曲屈服变形是十分有效的, 具有广泛的适用性。由于本文的泛最大有效力矩准则与郑亚东等的最大有效力矩准则设置的假定条件一样, 因此, 理论上该准则可以应用到任何因韧性弯曲屈服而发生变形的材料中, 应具有广阔的应用前景。
野外观测和高精度三维地震资料的构造解释均表明, 无论是韧性变形域还是脆性变形域, 出现共轭的现象是很少见的。在脆性变形域, coulomb-Mohr准则或Anderson断层模式[9]均预测出现共轭断层, 与自然界大量的断层作用现象不相容[18~21]。而根据先存构造活动性准则[22], 只有在均匀介质(如花岗岩), 或层状各向同性介质且最大主压应力与层面垂直(如水平地层中发生伸展作用)两种情况下, 才能出现共轭的脆性断裂, 其他任何非均匀介质(如包含有先存薄弱带)都不能出现共轭的脆性断裂。在韧性弯曲屈服变形中, 最大有效力矩准则预测出现共轭的变形带, 钝角(110°左右)对着最大主压应力方向。而根据泛最大有效力矩准则, 只有在先存面理平行或垂直最大主压应力方向时, 才能出现共轭的变形带, 其他方向的先存面理均不能出现共轭的变形带。这样, 一方面, 先存构造活动性准则和泛最大有效力矩准则, 很好地解释了自然界出现的脆性断裂和韧性变形带绝大部分是非共轭的现象, 另一方面表明脆性变形和韧性变形存在一定的共性。
5. 结论
导致变形带内先存面理或层理发生转动的最大有效力矩与先存面理或层理的方向有关, 可以用拓展后的最大有效力矩准则---泛最大有效力矩准则
当先存面理与σ1平行时, 在σ1左右两侧± 54.7°方向出现2个有效力矩的最大值(0.77 × 0.5(σ-σ3) L), 形成共轭的变形带, 钝角(109.4°)对着σ1方向; 当先存面理与σ1斜交时, 在σ1的另一侧出现1个有效力矩的最大值, 从而只出现一个方向的变形带, 并随着先存面理偏离σ1方向, 变形带与σ1的夹角逐渐减小(从θ=0°时的54.7°, 减小到θ=90°时的35.3°), 而与先存面理之间的夹角逐渐增大(从θ=0°时的54.7°, 增加到θ=90°时的125.3°); 当先存面理与σ1垂直时, 在σ1左右两侧± 35.3°方向出现2个有效力矩的最大值(0.77 × 0.5(σ1-σ3) L), 也形成共轭的变形带, 但锐角(70.6°)对着σ1方向。
在应力状态和先存面理力学性质一致的条件下，当先存面理与σ1呈45°夹角时(θ=45°)，最大有效力矩达到最大值(1.0×0.5(σ1-σ3)L)，沿潜在变形带(先存面理的垂直方向，α=-45°)发生变形相对最为容易; 当先存面理与σ1平行或垂直时(θ=0°或θ=90°)，最大有效力矩达到最小值(0.77×0.5(α1-σ3)L)，沿潜在变形带(两组共轭的变形带)发生变形相对最不容易。
通过在主应变平面上变形带与先存面理之间夹角的测量及变形带剪切方向(左旋或右旋)的确定，可以确定最大主压应力方向。
-
图 1 最大有效力矩准则力学分析示意图[1]
α-σ1与面理的夹角; θ-σ1与面理法线的夹角; H-有效力臂长度; L-σ1方向的单位长度
Figure 1. A sketch map of mechanical analysis of the Maximum Effective Moment Criterion
图 2 不同方位先存面理有效力矩计算参数示意图
AC的延伸方向为先存面理方向, 先存面理与σ1的夹角为θ(从σ1到先存面理, 逆时针为正), 变形带(BC方向)与σ1的夹角为α(从σ1到先存面理, 逆时针为正), AC为先存面理的单位长度L。单位长度先存面理沿变形带剪切弯曲的力臂为AB (H), 有效力矩为AB (H=Lsin (α-θ))与沿变形带剪应力(0.5(σ1-σ3) sin2α)的乘积(0.5(σ1-σ3) Lsin2αsin (α-θ))
Figure 2. Calculating parameters of effective moment of pre-exiting cleavages with different directions
图 3 不同方位先存面理沿不同方向的有效力矩分量sin2αsin (α-θ)的等值线图
a, b, c为不同方位先存面理sin2αsin (α-θ)值的极值分布线, 每一方位的先存面理都有3个极值点, 其中a中的A点(θ=90°)、b中的B点(θ=0°)以及c上的所有点(sin2αsin (α-θ))的绝对值都是最大值, 这样, 当θ=90°或θ=0°时, 有2个最大值, 其他的都只有1个最大值。图中数值的符号代表力矩的方向, 顺时针转动为正, 逆时针转动为负
Figure 3. Contour map of the effective moment component of pre-exiting cleavages with different directions
图 4 7个特征方位的先存面理的有效力矩分量sin2αsin(α-θ)与α变化关系及最大值分布图
Figure 4. Relationship between sin2αsin(α-θ) and α and the distributions of their maximum values
图 5 5个特征方位先存面理所对应的变形带及剪切方向关系图(虚线为先存面理方向)
Figure 5. Relationship between deformation zone and shear direction of different pre-exiting cleavages
图 6 利用先存面理与变形带间夹角ϕ及变形带剪切方向确定主压应力方位示意图
a-变形带左旋剪切, 从变形带起逆时针方向测量ϕ(为ϕa), 并计算确定α(αa), 从而确定σ1方向; b-变形带右旋剪切, 从变形带起顺时针方向测量ϕ(为ϕb), 并计算确定α(αb), 从而确定σ1方向; a和b中先存面理与变形带的方向一致, 但变形带的剪切方向不一致, 结果导致σ1方向相差90°(ϕb-ϕa=90°); 虚线为先存面理方向
Figure 6. Principle compressive stress direction determined by the angle ϕ and the shear direction of deformation zone
表 1 不同方位先存面理出现最大有效力矩的方位及其组合
Table 1. Directions and potential deformation zones of the maximum effective moment of the different cleavages
-
[1] ZHENG Ya-dong, WANG Tao, MA Ming-bo, et al.Maximum effective moment criterion and the origin of low-angle normal fault[J].Journal of Structural Geology, 2004, 26:271~285. doi: 10.1016/S0191-8141(03)00079-8 [2] 郑亚东, 王涛, 王新社.最大有效力矩准则的理论与实践[J].北京大学学报:自然科学版, 2007, 43(2):145~156. http://kns.cnki.net/KCMS/detail/detail.aspx?filename=bjdz200702000&dbname=CJFD&dbcode=CJFQZHENG Ya-dong, WNAG Tao, WANG Xin-she.Theory and practice of the Maximum Effective Moment Criterion (MEMC)[J].Acta Scientiarum Naturalium Universitatis Pekinensis, 2007, 43(2):145~156. http://kns.cnki.net/KCMS/detail/detail.aspx?filename=bjdz200702000&dbname=CJFD&dbcode=CJFQ [3] 郑亚东, 杜思清. 共轭扭折带夹角的定量分析[C]//马宗晋. 国际交流地质学术论文集---为二十七届国际地质大会撰写(2). 北京: 地质出版社, 1985: 187~190.ZHENG Ya-dong, DU Si-qing. A quantitative analysis of the angle between conjugate joint drags[C]//MA Zong-jin. Geological symposium for international interchange: Writing for the 27 th International Geological Conference (Ⅱ). Beijing: Geological Publishing House, 1985: 187~190. [4] ZHENG Ya-dong. A quantitative analysis of the angle between conjugate sets of extensional crenulation cleavage: An explanation of the low-angle normal fault development[C]//Abstract of 29 th IGC. 1992: 131. [5] Wernicke B.Low-angle normal faults in the Basin and Ridge Province:Nappe tectonics in an extending orogeny[J].Nature, 1981, 291:645~648. doi: 10.1038/291645a0 [6] Lister G S, Davis G A.The origin of metamorphic core complexes and detachment faults formed during Tertiary continental extension in the northern Colorado River region, USA[J].Journal of Structural Geology, 1989, 11(1):65~94. [7] Wernicke B, Burchfiel B C.Modes of extensional tectonics[J].Journal of Structural Geology, 1982, 4(1):105~115. [8] Wernicke B, Axen G J.On the role of isostasy in the evolution of normal fault systems[J].Geology, 1988, 16(9):848~851. doi: 10.1130/0091-7613(1988)016<0848:OTROII>2.3.CO;2 [9] Anderson E M.The dynamics of faulting:2 nd edition[M].Edingburgh:Oliver and Boyd, 1951. [10] Ramsay J G.Shear zone geometry:A review[J].Journal of Structural Geology, 1980, 2(1-2):83~99. doi: 10.1016/0191-8141(80)90038-3 [11] Paterson M S, Weiss L E.Experimental deformation and folding in phyllite[J].GSA Bulletin, 1966, 77(4):343~374. doi: 10.1130/0016-7606(1966)77[343:EDAFIP]2.0.CO;2 [12] 郑亚东, 张进江, 王涛.实践中发展的最大有效力矩准则[J].地质力学学报, 2009, 15(3):209~217. http://journal.geomech.ac.cn/ch/reader/view_abstract.aspx?flag=1&file_no=20090301&journal_id=dzlxxbZHENG Ya-dong, ZHANG Jin-jiang, WANG Tao.The Maximum-Effective-Moment Criterion developing in practice[J].Journal of Geomechanics, 2009, 15(3):209~217. http://journal.geomech.ac.cn/ch/reader/view_abstract.aspx?flag=1&file_no=20090301&journal_id=dzlxxb [13] Hill R.The mathematical theory of plasticity[M].Oxford:Oxford University Press, 1950. [14] Platt J P, Vissers R L M.Extensional structures in anisotropic rocks[J].Journal of Structural Geology, 1980, 2(4):397~410. doi: 10.1016/0191-8141(80)90002-4 [15] Price N J, Cosgrave J W.Analysis of geological structures[M].Cambridge:Cambridge University Press, 1990. [16] Johnson A M.Styles of folding:Mechanics and mechanisms of folding of natural elastic materials[M].New York:Elservier Scientific Publication Company, 1977. [17] Camerlo R H, Benson E F.Geometric and seismic interpretation of the Perdido fold belt:Northwestern deep-water Gulf of Mexico[J].AAPG Bulletin, 2006, 90(3):363~386. doi: 10.1306/10120505003 [18] 童亨茂, 孟令箭, 蔡东升, 等.裂陷盆地断层的形成和演化---目标砂箱模拟实验与认识[J].地质学报, 2009, 83(6):759~774. http://kns.cnki.net/KCMS/detail/detail.aspx?filename=dzxe200906003&dbname=CJFD&dbcode=CJFQTONG Heng-mao, MENG Ling-jian, CAI Dong-sheng, et al.Fault formation and evolution in rift basins:Sandbox modeling and cognition[J].Acta Geologica Sinica, 2009, 83(6):759~774. http://kns.cnki.net/KCMS/detail/detail.aspx?filename=dzxe200906003&dbname=CJFD&dbcode=CJFQ [19] Reches Z.Analysis of faulting in three-dimensional strain field[J].Tectonophysics, 1978, 47(1-2):109-129. doi: 10.1016/0040-1951(78)90154-3 [20] Krantz R W.Multiple fault sets and three-dimensional strain:Theory and application[J].Journal of Structural Geology, 1988, 10(3):225~237. doi: 10.1016/0191-8141(88)90056-9 [21] Nieto-Samaniego, ángel F.Stress, strain and fault patterns[J].Journal of Structural Geology, 1999, 21(8-9):1065~1070. doi: 10.1016/S0191-8141(99)00016-4 [22] TONG Heng-mao, CAI Dong-sheng, WU Yong-ping, et al.Activity criterion of pre-existing fabrics in non-homogeneous deformation domain[J].Science China:Earth Science, 2010, 53(1):1~11. -
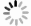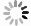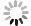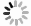
下载:
下载:
计量
- 文章访问数: 189
- HTML全文浏览量: 61
- PDF下载量: 11
- 被引次数: 0
